Back
Problem 1
Two solids are shown below. One is a semiconductor and one is an insulator. Which one is which? Explain your reasoning.
Problem 2
For each of the two-dimensional structures shown here (a) draw the unit cell (i)
(ii)
Problem 3
Shown here are sketches of two processes. Which of the processes refers to the ductility of metals and which refers to malleability of metals? (a)
(b)
Problem 5b
(b) What is the coordination number of each cannonball in the interior of the stack?
Problem 6
Which arrangement of cations (yellow) and anions (blue) in a lattice is the more stable? Explain your reasoning. (a)
(b)
Problem 7
Which of these molecular fragments would you expect to be more likely to give rise to electrical conductivity? Explain your reasoning. (a)
(b)
Problem 8c
The electronic structure of a doped semiconductor is shown here. (c) Which region of the diagram represents the band gap?
Problem 9b
Shown here are cartoons of two different polymers. Which one would have the higher melting point?
Problem 10a
The accompanying image shows photoluminescence from four different samples of CdTe nanocrystals, each embedded in a polymer matrix. The photoluminescence occurs because the samples are being irradiated by a UV light source. The nanocrystals in each vial have different average sizes. The sizes are 4.0, 3.5, 3.2, and 2.8 nm. (a) Which vial contains the 4.0-nm nanocrystals?
Problem 12a
Silicon is the fundamental component of integrated circuits. Si has the same structure as diamond. (a) Is Si a molecular, metallic, ionic, or covalent-network solid?
Problem 12b
Silicon is the fundamental component of integrated circuits. Si has the same structure as diamond. (b) Silicon readily reacts to form silicon dioxide, SiO2, which is quite hard and is insoluble in water. Is SiO2 most likely a molecular, metallic, ionic, or covalent-network solid?
Problem 13a
What kinds of attractive forces exist between particles (atoms, molecules, or ions) in (a) molecular crystals?
Problem 13d
What kinds of attractive forces exist between particles (atoms, molecules, or ions) in (d) and metallic crystals?
Problem 14a
Which type (or types) of crystalline solid is characterized by each of the following? (a) High mobility of electrons throughout the solid;
Problem 14b
Which type (or types) of crystalline solid is characterized by each of the following? (b) softness, relatively low melting point;
Problem 14c
Which type (or types) of crystalline solid is characterized by each of the following? (c) high melting point and poor electrical conductivity;
Problem 14d
Which type (or types) of crystalline solid is characterized by each of the following? (d) network of covalent bonds.
Problem 15c
Indicate the type of solid (molecular, metallic, ionic, or covalent-network) for each compound: (c) Ta2O5 (melting point, 1872°C)
Problem 17
You are given a gray substance that melts at 700 °C; the solid is a conductor of electricity and is insoluble in water. Which type of solid (molecular, metallic, covalent-network, or ionic) might this substance be?
Problem 19a
(a) Draw a picture that represents a crystalline solid at the atomic level.
Problem 19b
(b) Now draw a picture that represents an amorphous solid at the atomic level.
Problem 21b
Two patterns of packing for two different circles of the same size are shown here. For each structure (b) determine the angle between the lattice vectors, g, and determine whether the lattice vectors are of the same length or of different lengths; (i)
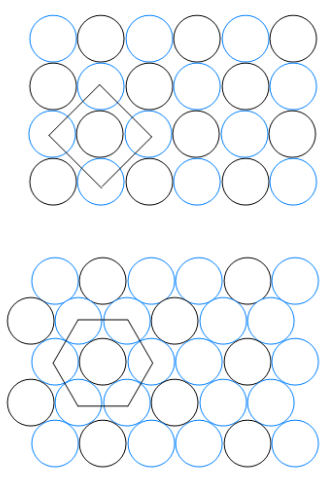
(ii)
Problem 22c
Two patterns of packing two different circles of the same size are shown here. For each structure (c) determine the type of two-dimensional lattice (from Figure 12.4). (i)
(ii)
Problem 23
Imagine the primitive cubic lattice. Now imagine grabbing the top of it and stretching it straight up. All angles remain 90. What kind of primitive lattice have you made?
Problem 25
Which of the three-dimensional primitive lattices has a unit cell where none of the internal angles is 90? (a) Orthorhombic, (b) hexagonal, (c) rhombohedral, (d) triclinic, (e) both rhombohedral and triclinic.
Problem 28
What is the minimum number of atoms that could be contained in the unit cell of an element with a face-centered cubic lattice? (a) 1, (b) 2, (c) 3, (d) 4, (e) 5.
Problem 29b
The unit cell of nickel arsenide is shown here. (b) What is the empirical formula?
Problem 30a
The unit cell of a compound containing potassium, aluminum, and fluorine is shown here. (a) What type of lattice does this crystal possess (all three lattice vectors are mutually perpendicular)?
Problem 33a
Consider the unit cells shown here for three different structures that are commonly observed for metallic elements. (a) Which structure(s) corresponds to the densest packing of atoms?
Problem 33b
Consider the unit cells shown here for three different structures that are commonly observed for metallic elements. (b) Which structure(s) corresponds to the least dense packing of atoms?
