Textbook Question
Two solids are shown below. One is a semiconductor and one is an insulator. Which one is which? Explain your reasoning.
337
views

 Verified step by step guidance
Verified step by step guidance


Two solids are shown below. One is a semiconductor and one is an insulator. Which one is which? Explain your reasoning.
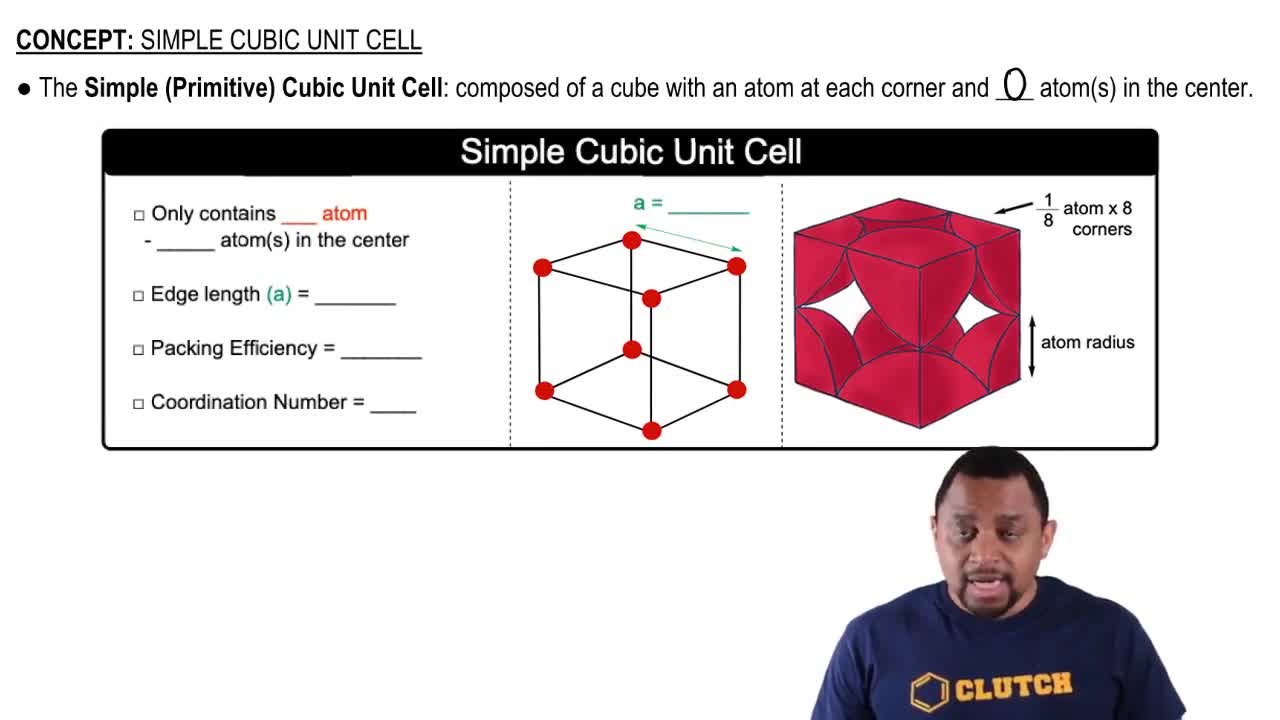
For each of the two-dimensional structures shown here (a) draw the unit cell (i)
(ii)
Shown here are sketches of two processes. Which of the processes refers to the ductility of metals and which refers to malleability of metals? (a)
(b)
Which arrangement of cations (yellow) and anions (blue) in a lattice is the more stable? Explain your reasoning. (a)
(b)
Which of these molecular fragments would you expect to be more likely to give rise to electrical conductivity? Explain your reasoning. (a)
(b)
The electronic structure of a doped semiconductor is shown here. (c) Which region of the diagram represents the band gap?