Molarity - Online Tutor, Practice Problems & Exam Prep

Molarity (M) is a specific measure of concentration, defined as the number of moles of solute per liter of solution. The formula for molarity is M=moles of soluteliters of solution. Understanding molarity allows for the calculation of unknown quantities in solutions, utilizing conversion factors that connect different units, such as in a 5.8 M sodium chloride solution, which indicates 5.8 moles of NaCl per liter of solution.

Molarity

In these series of videos, we're going to take a look at the concept of molarity. Now molarity itself is represented by the variable m. And in class, you'll hear molarity and concentration sometimes being used interchangeably. Remember, concentration represents the amount of a given solute within a certain amount of solution. Now molarity is more specific. Molarity represents the number of moles of solute per liter of solution, and with this, we have our molarity formula. So molarity, which is capital M, equals moles of solute over liters of solution.

M = moles of solute liters of solution

Now that we have a more fleshed out idea of what concentration is through molarity, let's take a look at some problems or ask to calculate molarity or to look at the components that help to make molarity.

Molarity Example 1

Here it says to calculate the molarity of a solution prepared by dissolving 23.7 grams of sodium hydroxide in enough water to make 2.50 liters of solution. So here we're looking for molarity. Molarity here equals our moles of solute which would be the NaOH and it is in a solution of 2.50 liters. So we need liters of solution. Alright. We already have the 2.50 liters of solution, so we just need to convert the grams of sodium hydroxide into moles. One mole of sodium hydroxide goes on the bottom and then grams of sodium hydroxide go on the bottom. Sodium hydroxide is composed of 1 sodium, 1 oxygen, and 1 hydrogen. Multiply them by their atomic masses from the periodic table, and then add up the totals. This will give us the molar mass of sodium hydroxide.

Molar mass of NaOH = 39.998 g

So that's gonna go here. Here, grams of sodium hydroxide cancel out, and now I'm gonna have moles of sodium hydroxide. So that comes out to 0.5925 moles. Take that and plug it in, and we'll have the molarity of our solution:

Molarity = 0.5925 moles of NaOH / 2.50 liters of solution = 0.237  molar

So this comes out to 0.237 molar for our solution.

Molarity

Now that we know what molarity is, we can use it to help us calculate unknowns. We're going to say problems with a molarity value present can use a given amount and conversion factors to isolate an end amount. If you haven't watched our videos on dimensional analysis and conversion factors, I highly suggest you go back and take a look. Because it's just a continuation of this. Now we are incorporating molarity into our calculations. The molarity value itself can represent one of those conversion factors because remember, conversion factors possess two different units connected together. For example, we say 5.8 molar sodium chloride. 5.8 molar represents 5.8 moles of NaCl within 1 liter of solution. And because we're using two different units connected together, this is a conversion factor. So we can write it as 5.8moles of NaCl1 liter of solution. This is what 5.8 molar really represents. So keep that in mind when we start doing calculations.

Molarity Example 2

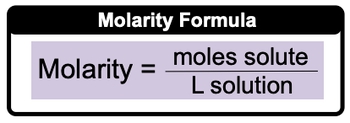
In this example question, it asks how many grams of sodium phosphate, which has a molecular mass of 163.94 grams per mole, are present in 300 milliliters of a 0.550 molar sodium phosphate solution. Now, before we start plugging in numbers and doing calculations, let's discuss what the sentence indicates and how we should interpret it when it's presented in such a way. We have several numbers, connected by the word "of". Remember, when "of" joins two values, it typically instructs us to multiply them together. Here, we encounter molarity, which is defined as moles over liters. If we multiply both sides by liters, it shows that moles equal liters times molarity.

Notice that the volume provided is in milliliters. To proceed, we need to convert milliliters to liters and then multiply by the molarity to determine the moles of sodium phosphate. Knowing the moles of a compound allows us to use its molecular mass to calculate the grams of that compound. We start by converting 300 milliliters into liters. This is a metric prefix conversion, where milliliters are converted to liters using the factor 1milli=10-3. Milliliters cancel out, leaving us with liters.

Molarity also acts as a conversion factor. The 0.550 molar indicates we have 0.550 moles of the compound per 1 liter. We need liters to cancel out, so we place 1 liter on the opposite side, resulting in an expression that just leaves moles of sodium phosphate. Converting these moles to grams, we use the given molecular weight of 163.94 grams per mole. Thus, the final step involves multiplying the moles by the molecular weight. Initially, this gives us 27.0501 grams of sodium phosphate.

Considering significant figures, 300.0 has four, 0.550 has three, and 163.94 has five. We use the least number of significant figures for our final answer, yielding 27.1 grams of sodium phosphate. So, when the problem gives us a volume of molarity, it essentially provides the moles. In this case, we simply converted those moles to grams to find the answer.

What volume in (µL) of 0.125 M HBr contains 0.170 moles HBr?

• A. 7.35x10³ μ L
• B. 7.35x10⁶ μ L
• C. 1.36x10³ μ L
• D. 1.36x10⁹ μ L
• E. 1.36x10⁶ μ L

Hypernatremia is a medical condition where a patient has high levels of sodium in their blood, and is the result of the body containing too little water. A patient has a measured sodium level of 165 mM. If 30.0 mL of their blood were drawn, what mass (in ng) of sodium would be present?

• A. 1.14x10⁷ ng
• B. 1.14x10⁶ ng
• C. 1.14x10⁸ ng
• D. 4.18x10⁸ ng
• E. 4.18x10⁶ ng

2.64 grams of an unknown compound was dissolved in water to yield 150 mL of solution. The concentration of the solution was 0.075 M. What was the molecular weight of the substance?

• A. 190 g/mol
• B. 30 g/mol
• C. 90 g/mol
• D. 230 g/mol
• E. 320 g/mol

A solution with a final volume of 750.0 mL was prepared by dissolving 30.00 mL of benzene (C₆H₆, density = 0.8787 g/mL) in dichloromethane. Calculate the molarity of benzene in the solution.

• A. 0.45 M
• B. 0.035 M
• C. 0.35 M
• D. 0.71 M
• E. 0.091 M

Here’s what students ask on this topic:

What is the formula for calculating molarity?

The formula for calculating molarity (M) is:

M=moles of soluteliters of solution

This formula indicates that molarity is the number of moles of solute divided by the volume of the solution in liters. For example, if you have 1 mole of solute dissolved in 1 liter of solution, the molarity is 1 M.

How do you calculate the molarity of a solution?

To calculate the molarity of a solution, follow these steps:

1. Determine the number of moles of solute present in the solution.
2. Measure the volume of the solution in liters.
3. Use the molarity formula:

M=moles of soluteliters of solution

For example, if you have 2 moles of solute in 1 liter of solution, the molarity is:

M=2 moles1 liter=2 M

What is the difference between molarity and concentration?

While molarity and concentration are often used interchangeably, they have specific meanings. Concentration is a general term that describes the amount of solute in a given amount of solution. Molarity, on the other hand, is a specific measure of concentration defined as the number of moles of solute per liter of solution. The formula for molarity is:

M=moles of soluteliters of solution

Thus, molarity is a more precise way to express concentration, providing a clear relationship between the amount of solute and the volume of the solution.

How can molarity be used as a conversion factor in calculations?

Molarity can be used as a conversion factor because it connects two different units: moles of solute and liters of solution. For example, if you have a 5.8 M solution of NaCl, this means there are 5.8 moles of NaCl per liter of solution. You can write this as a conversion factor:

5.8 moles NaCl1 liter solution

This allows you to convert between moles of solute and volume of solution in various calculations. For instance, if you need to find out how many moles of NaCl are in 2 liters of a 5.8 M solution, you can multiply:

5.8 moles NaCl × 2 liters = 11.6 moles NaCl

What are some common mistakes to avoid when calculating molarity?

When calculating molarity, avoid these common mistakes:

1. Not converting units: Ensure the volume is in liters and the amount of solute is in moles.
2. Incorrectly measuring volume: Use accurate tools to measure the solution volume.
3. Forgetting to account for dilution: If the solution is diluted, recalculate the molarity.
4. Misinterpreting the formula: Remember, molarity is moles of solute per liter of solution, not per liter of solvent.

By being mindful of these points, you can accurately calculate molarity and avoid errors in your results.