The Ideal Gas Law: Molar Mass - Online Tutor, Practice Problems & Exam Prep

Understanding the molar mass of a gas is crucial in chemistry, and the ideal gas law provides a method to calculate it. Molar mass (M) is the mass of a substance (in grams) divided by the amount of substance (in moles). The ideal gas law can be rearranged to find the molar mass of a gas using the formula:

$M=\frac{mRT}{PV}$

where m is the mass of the gas in grams, R is the ideal gas constant, T is the temperature in Kelvin, P is the pressure, and V is the volume. This formula allows for the direct calculation of molar mass from the properties of a gas, streamlining the process and eliminating the need for step-by-step calculations. It's a powerful tool for chemists to determine the molar mass from experimental gas data, providing insight into the molecular composition and behavior of gases.

The Ideal Gas Law: Molar Mass

Up to this point we've used the ideal gas law to help us isolate pressure, volume, moles or temperature. But now we're going to be faced with a new but old topic, the idea of molar mass. Now recall molar mass represents the mass of a substance divided by the amount of that substance.

If we take a look here, we're going to say that this capital M represents the molar mass of the gas and lower case m here equals the mass of the gas in grams, and N we know from the ideal gas law equals the amount of the gas in moles, so molar mass equals grams per mole. Now that we remember what molar mass is, we're going to take a look at how we can use the ideal gas law to find the molar mass of a gas South.

For now, click on the next video and let's take a look at an example question.

The Ideal Gas Law: Molar Mass Example 1

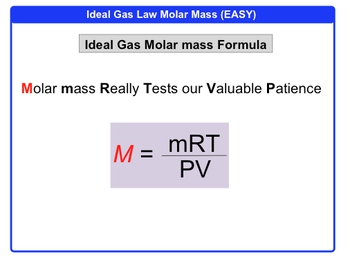
Here we're told calculate the molar mass of a gas if 2.50g occupies 0.995 liters at 715 Tor and 40�C. All right, we need to calculate molar mass. OK, now, molar mass here equals grams over moles. So let's just say that grams over moles within the question, they give us grams. Right off the bat, we have 2.50 grams, but we don't have the moles.

However, in the question we see that we have volume, we have pressure, and we have temperature. V&T, we know are part of the ideal gas law, so we're going to say PV=nRT. With this information we can isolate the moles of our gas. So divide both sides here by RT and we're going to say here that moles equals pressure times volume over RT. We need to convert our pressure into atmospheres.

So remember we have 715 tours and then we're going to stay here. For every one atmosphere that we have that's equal to 760 tours, tours here would cancel out and we get as our pressure 0.9408 atmospheres. Take those atmospheres and plug them in. So we have 0.9408 atmospheres. Our volume is already in liters, so we're good there. Remember R is our gas constant which is 0.08206 liters times atmospheres over moles times K and temperature.

Temperature has to be in Kelvin O. Add 273.15 to this. So when we do that, that's going to give us 313.15 Kelvin. Plug that in. What units? Cancel out, Atmospheres cancel out, liters cancel out, Kelvin's cancel out. And that's how we see we'll have moles at the end. So here when we do that, we get our moles as 0.0364 moles, take those moles and plug them into the molar mass formula, and doing that gives us a value of 68.681g per mole.

Now here 2.50 has three sig figs, 3 sig figs, 3 sig figs, 40 has only one sig fig. So if we wanted to go by that one sig fig, that will come out to 70 grams per mole. It's not as accurate, but it's not a big deviation from our initial answer. So if we're going by significant figures, we can say that 70 grams per mole would be the molar mass of our unknown gas.

The Ideal Gas Law: Molar Mass

Besides finding the moles of a gas sample, the ideal gas law can be extended further to find the molar mass of a gas. Here we're going to look at the easy way on how we were able to derive the ideal gas law to this new version. Besides this easy method, we have a harder method. You can click on the next video to see how I derived this formula, but again, only look at that video if your professor cares on showing how you derive this new version of the ideal gas law.

If your professor doesn't care, then just ignore that video and justice go on to the next question. What we have to solve for a problem. All right. So here to help us remember this one, we're going to say more mass really tests our valuable patience. So here molar and then mass really tests RT. They are over valuable patients, which is volume and pressure. Here I just kept them in the order of PV.

So this is the formula we can utilize to find the molar mass of a gas immediately instead of doing it piece by piece like we did in previous example questions. So just remember, molar mass really tests our valuable patience M=mRT/PV. So now that we've seen this easy method, you can click on to the next video to look at how I derived this formula, or you can skip that video and just head straight to questions or utilize this particular newly derived ideal gas law formula.

The Ideal Gas Law: Molar Mass

So for those of you who clicked on this video, let's see how we derived this new version of the ideal gas law. So we start out with the molar mass formula. Remember, molar mass equals mass M divided by moles N. Here we're going to algebraically rearrange this formula to isolate N. So first you're going to multiply both sides by N. So now it becomes M times N equals lowercase m.

To isolate N and moles, we divide out molar mass. So we're going to say here moles equals mass divided by molar mass. Going to the ideal gas law formula, we can now substitute N for this value here. So PV equals NRT now becomes PV equals mass over molar mass. We need to isolate our molar mass.

So what I'm going to do here is I'm going to multiply both sides by molar mass. Here is a denominator so I can multiply to get rid of it, and that equals mass times RT. Finally, to isolate our molar mass, we divide both sides by PV, and that's how we got that molar mass equals MRT/PV.

Again, this is the way we derived it if your professor wants you to show the work. But again, it's always easier to remember that molar mass really tests our valuable patience. Remembering that will help you remember this version of the ideal gas law.

The Ideal Gas Law: Molar Mass Example 2

Here it states in this example question, an unknown gas with a mass of 0.1727 grams is placed into 125 milliliter flask if its pressure is 0.833 atmospheres at 20�C. What is the identity of the gas here? We're given different gases and we need to realize here that they're giving us our grams, which is Massachusetts. They're giving us volume, they're giving us pressure, and they're giving us temperature.

With this, we'll be able to find the molar mass of our unknown gas, and then all we have to do is see which one has that molar mass as their actual weight. So we're going to say here molar mass equals RTPV. We're going to plug in the mass of our unknown. We're going to plug in R, which is 0.08206 liters times atmospheres over moles times K. Remember, temperature has to be in Kelvin, so add 273.15 to this number to give us 293.15 K divided by our pressure, which is 0.833 atmospheres and then our volume.

We convert those milliliters into liters, so that gives us 0.125 liters. Here we look and see what units cancel out and we see all that's left is grams over moles, so we have molar mass. When you plug that in, you get approximately 39.90g per mole. All we have to do now is see which one of these gases has a molar mass closest to our answer.

So here N₂, the two nitrogens, comes out to 28.02 grams per mole. Argon, from the periodic table, is approximately 39.95 grams per mole. Oxygen is 32g per mole. Neon is approximately 20 or so grams per mole according to the periodic table. And then chapter 4 is roughly around 16g per mole. Our closest answer would have to be argon. It's the one that's closest to this 39.90g per mole.

So here we've just utilized the molar mass version of the ideal gas law to find the molar mass of our unknown gas.

To identify a homonuclear diatomic gas, a chemist weighted an evacuated flask with a volume of 3.9 L then filled it with the gas at a pressure of 2.00 atm and 29.0 ºC. The chemist then re-weighted the flask and recorded the difference in mass as 8.81 g. Identify the gas.

• A. H₂
• B. N₂
• C. Cl₂
• D. F₂
• E. O₂

What is the molecular formula of a compound that contains 39.0% carbon, 16.0% hydrogen, and 45.0% nitrogen, if 0.1576 g of the compound occupies 125 mL with a pressure of 0.9820 atm at 295.15 K?

• A. CH₃N
• B. CH₅N
• C. C₂H₃N₂
• D. C₂H₅N₂

Here’s what students ask on this topic:

How can molar mass be found using the ideal gas law?

To find the molar mass using the Ideal Gas Law, you first need to know the other variables in the equation PV=nRT, where P is pressure, V is volume, n is the number of moles, R is the ideal gas constant, and T is temperature.

Assuming you have the values for pressure, volume, and temperature, and you know the amount of gas in grams (m), you can rearrange the Ideal Gas Law to solve for n (moles), which is m (mass of the gas in grams) divided by M (molar mass of the gas in grams per mole):

n=PVRT

Now, to find the molar mass (M), rearrange the equation to solve for M:

M=mRTPV

Here's a step-by-step guide:

1. Measure the mass of the gas in grams (m).
2. Measure the volume (V) of the gas.
3. Measure the temperature (T) in Kelvin.
4. Measure the pressure (P) of the gas.
5. Use the ideal gas constant (R) in the appropriate units to match your pressure and volume units.
6. Plug these values into the rearranged Ideal Gas Law equation to solve for the molar mass (M).

Remember to keep your units consistent, especially for temperature (which should be in Kelvin).

How can I find molar mass using the ideal gas law?

To find the molar mass using the ideal gas law, you'll need to rearrange the ideal gas law formula to solve for molar mass. The ideal gas law is:

P V = n R T

where P is pressure, V is volume, n is the number of moles, R is the ideal gas constant, and T is temperature in Kelvin.

First, you need to isolate n (the number of moles) by rearranging the equation:

n = P V R T

Then, remember that molar mass (M) is the mass (m) of the gas divided by the number of moles (n):

M = m n

Combine these two relationships by substituting n from the ideal gas law:

M = m P V R T

Now you have a formula for molar mass that incorporates the ideal gas law:

M = m R T P V

To find the molar mass, you'll need to know the mass of the gas sample (m), the pressure (P), the volume (V), and the temperature (T). Insert these values into the formula, and use the appropriate value for R (8.314 J/(mol·K) for SI units), and you can calculate the molar mass of the gas.