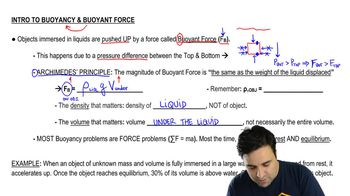
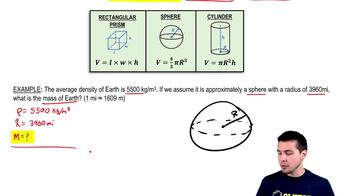
Textbook Question
Hydraulic Lift II.The piston of a hydraulic automobile lift is 0.30 m in diameter. What gauge pressure, in pascals, is required to lift a car with a mass of 1200 kg? Also express this pressure in atmospheres.
910
views
1
rank

 Verified step by step guidance
Verified step by step guidance