Here are the essential concepts you must grasp in order to answer the question correctly.
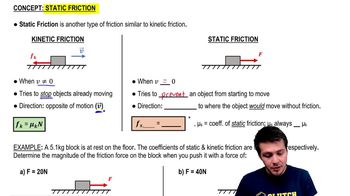
Friction
Friction is the force that opposes the relative motion of two surfaces in contact. It is dependent on the nature of the surfaces and the normal force acting between them. The static friction force must be overcome to initiate motion, while kinetic friction acts on moving objects. The coefficients of static and kinetic friction are crucial for calculating the forces required to move an object.
Recommended video:
Static Friction & Equilibrium
Newton's Second Law of Motion
Newton's Second Law states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. This relationship is expressed by the equation F = ma, where F is the net force, m is the mass, and a is the acceleration. Understanding this law is essential for analyzing how forces affect the motion of objects, especially in different gravitational environments.
Recommended video:
Intro to Forces & Newton's Second Law
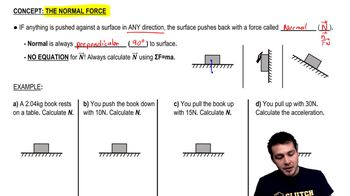
Weight and Normal Force
Weight is the force exerted by gravity on an object, calculated as the product of its mass and the acceleration due to gravity (W = mg). The normal force is the support force exerted by a surface perpendicular to the object resting on it. On the Moon, where gravity is weaker, the weight of the crate is less, affecting the normal force and consequently the frictional forces that must be overcome to initiate and maintain motion.
Recommended video: