A 550-N physics student stands on a bathroom scale in an elevator that is supported by a cable. The combined mass of student plus elevator is 850 kg. As the elevator starts moving, the scale reads 450 N. (a) Find the acceleration of the elevator (magnitude and direction).
When jumping straight up from a crouched position, an average person can reach a maximum height of about 60 cm. During the jump, the person's body from the knees up typically rises a distance of around 50 cm. To keep the calculations simple and yet get a reasonable result, assume that the entire body rises this much during the jump. (c) In terms of this jumper's weight w, what force does the ground exert on him or her during the jump?
 Verified step by step guidance
Verified step by step guidance
Verified video answer for a similar problem:
Key Concepts
Newton's Second Law of Motion

Gravitational Force

Kinematics of Vertical Motion
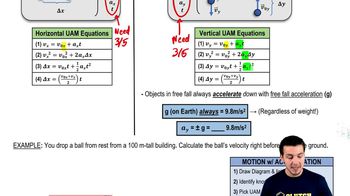
When jumping straight up from a crouched position, an average person can reach a maximum height of about 60 cm. During the jump, the person's body from the knees up typically rises a distance of around 50 cm. To keep the calculations simple and yet get a reasonable result, assume that the entire body rises this much during the jump. (a) With what initial speed does the person leave the ground to reach a height of 60 cm?
When jumping straight up from a crouched position, an average person can reach a maximum height of about 60 cm. During the jump, the person's body from the knees up typically rises a distance of around 50 cm. To keep the calculations simple and yet get a reasonable result, assume that the entire body rises this much during the jump. (b) Draw a free-body diagram of the person during the jump.
A 2.00-kg box is moving to the right with speed 9.00 m/s on a horizontal, frictionless surface. At t = 0 a horizontal force is applied to the box. The force is directed to the left and has magnitude F(t) = (6.00 N/s2)t2. (a) What distance does the box move from its position at t = 0 before its speed is reduced to zero?
A 5.00-kg crate is suspended from the end of a short vertical rope of negligible mass. An upward force F(t) is applied to the end of the rope, and the height of the crate above its initial position is given by y(t) = (2.80 m/s)t + (0.610 m/s3)t3. What is the magnitude of F when t = 4.00 s?
In a laboratory experiment on friction, a 135-N block resting on a rough horizontal table is pulled by a horizontal wire. The pull gradually increases until the block begins to move and continues to increase thereafter. Figure E5.26 shows a graph of the friction force on this block as a function of the pull. (a) Identify the regions of the graph where static friction and kinetic friction occur.
