Here are the essential concepts you must grasp in order to answer the question correctly.
Acceleration
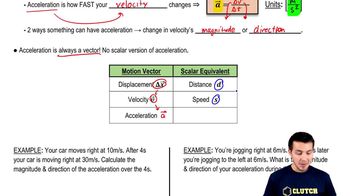
Acceleration is the rate of change of velocity of an object over time. It is a vector quantity, meaning it has both magnitude and direction. In the context of the question, the acceleration experienced during a sudden stop is crucial, as it determines whether the forces acting on the body exceed the survivable limit of 250 m/s².
Recommended video:
Kinematics
Kinematics is the branch of mechanics that deals with the motion of objects without considering the forces that cause the motion. It involves equations that relate displacement, velocity, acceleration, and time. To solve the problem, kinematic equations can be used to find the distance over which the airbag must stop the occupant safely.
Recommended video:
Impulse and Momentum
Impulse is the change in momentum of an object when a force is applied over a period of time. Momentum is the product of an object's mass and its velocity. In a crash scenario, the airbag provides an impulse that reduces the occupant's momentum to zero, and understanding this relationship is essential to calculate the stopping distance required to ensure the acceleration remains within survivable limits.
Recommended video:
Impulse & Impulse-Momentum Theorem