Here are the essential concepts you must grasp in order to answer the question correctly.
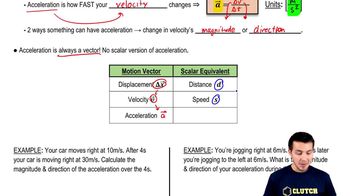
Acceleration
Acceleration is the rate of change of velocity of an object with respect to time. In this scenario, the rocket experiences a time-dependent acceleration given by ay = (2.80 m/s³)t, meaning that the acceleration increases linearly with time. Understanding how acceleration affects velocity is crucial for determining the rocket's speed at a specific height.
Recommended video:
Kinematics Equations
Kinematics equations describe the motion of objects under constant or variable acceleration. These equations relate displacement, initial velocity, final velocity, acceleration, and time. In this problem, we will need to integrate the acceleration function to find the velocity as a function of time, which is essential for calculating the speed of the rocket at a height of 325 m.
Recommended video:
Integration
Integration is a mathematical process used to find the accumulated value of a function over an interval. In the context of this problem, we need to integrate the acceleration function to obtain the velocity function. This step is necessary to determine how the rocket's speed changes over time, allowing us to find the speed at the specified height.
Recommended video:
Finding Moment Of Inertia By Integrating
 Verified step by step guidance
Verified step by step guidance