Here are the essential concepts you must grasp in order to answer the question correctly.
Velocity
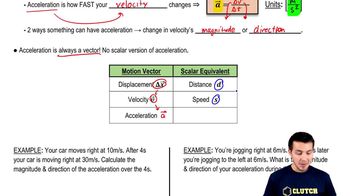
Velocity is a vector quantity that describes the rate of change of an object's position with respect to time. It has both magnitude and direction, typically expressed in meters per second (m/s). In the context of the question, the velocity of the cat is represented on the y-axis of the graph, indicating how fast and in which direction the cat is moving along the x-axis.
Recommended video:
Acceleration
Acceleration is the rate of change of velocity over time, measured in meters per second squared (m/s²). It indicates how quickly an object is speeding up or slowing down. In the given scenario, the acceleration can be determined from the slope of the velocity-time graph; a constant slope indicates uniform acceleration, while a changing slope would indicate varying acceleration.
Recommended video:
Slope of a Graph
The slope of a graph represents the relationship between two variables. In a velocity-time graph, the slope corresponds to acceleration. A positive slope indicates that the object is accelerating, while a negative slope indicates deceleration. The steeper the slope, the greater the acceleration. In this case, the linear increase in the velocity graph suggests a constant positive acceleration for the cat.
Recommended video: