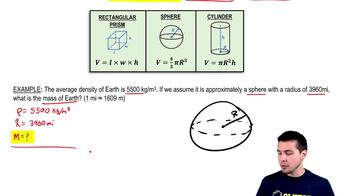
Here are the essential concepts you must grasp in order to answer the question correctly.
Mass Density
Mass density is defined as the mass of a substance per unit volume, typically expressed in kilograms per cubic meter (kg/m³). It is a crucial property that indicates how much mass is contained in a given volume of material. In the context of gases, density can change with variations in pressure and temperature, but for an ideal gas, it is directly proportional to the number of moles and inversely proportional to volume.
Recommended video:
Problems with Mass, Volume, & Density
Ideal Gas Law
The Ideal Gas Law is a fundamental equation in thermodynamics that relates the pressure, volume, temperature, and number of moles of an ideal gas. It is expressed as PV = nRT, where P is pressure, V is volume, n is the number of moles, R is the ideal gas constant, and T is temperature. This law helps in understanding how changes in volume and pressure affect the behavior of gases, particularly during processes like compression.
Recommended video:
Ideal Gases and the Ideal Gas Law
Compression of Gases
Compression of gases involves reducing the volume of a gas, which typically increases its pressure and can affect its temperature. According to Boyle's Law, for a given amount of gas at constant temperature, the pressure of the gas is inversely proportional to its volume. When the volume is halved, the density of the gas increases, as density is mass divided by volume, leading to a higher mass density if the mass remains constant.
Recommended video:
Internal Energy of Ideal Monoatomic Gases