Here are the essential concepts you must grasp in order to answer the question correctly.
Center of Mass
The center of mass is the point at which the mass of an object is considered to be concentrated. For uniform bricks, the center of mass is located at their geometric center. When stacking bricks, the position of the center of mass relative to the base of the stack is crucial for stability; if the center of mass extends beyond the edge of the base, the stack will topple.
Recommended video:
Torque and Equilibrium
Torque is the rotational equivalent of linear force and is calculated as the product of force and the distance from the pivot point. For the stack of bricks to remain in equilibrium, the sum of torques around the pivot point (the edge of the table) must be zero. This means that the clockwise torques must balance the counterclockwise torques, which is essential for determining how far the top brick can extend without causing the stack to fall.
Recommended video:
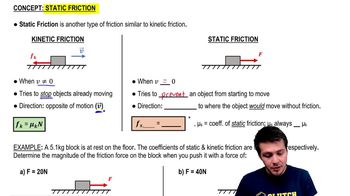
Static Friction
Static friction is the force that prevents two surfaces from sliding past each other when at rest. In the context of stacking bricks, static friction between the bricks and the table helps to maintain the stability of the stack. Understanding the role of static friction is important for analyzing how the bricks can be positioned without slipping, which directly affects the maximum distance the top brick can extend.
Recommended video:
Static Friction & Equilibrium
 Verified step by step guidance
Verified step by step guidance