Here are the essential concepts you must grasp in order to answer the question correctly.
Moment of Inertia
The moment of inertia is a measure of an object's resistance to rotational motion about a specific axis. It depends on the mass distribution relative to that axis, calculated as the sum of the products of each mass and the square of its distance from the axis. For a system of particles, it is expressed as I = Σ(m_i * r_i^2), where m_i is the mass and r_i is the distance from the axis of rotation.
Recommended video:
Intro to Moment of Inertia
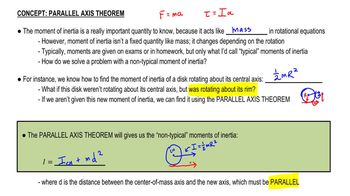
Parallel Axis Theorem
The parallel axis theorem allows for the calculation of the moment of inertia of a body about any axis, given its moment of inertia about a parallel axis through its center of mass. It states that I = I_cm + Md^2, where I_cm is the moment of inertia about the center of mass, M is the total mass, and d is the distance between the two axes. This theorem is essential when dealing with complex systems of masses.
Recommended video:
Rigid Body Dynamics
Rigid body dynamics studies the motion of solid objects that do not deform under stress. In this context, the masses connected by massless rods can be treated as a rigid body, allowing for the application of rotational motion principles. Understanding how forces and torques affect the motion of such systems is crucial for calculating quantities like the moment of inertia and analyzing their rotational behavior.
Recommended video: