Textbook Question
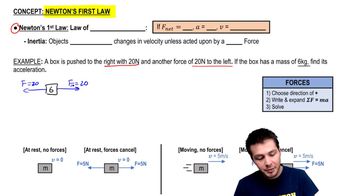
Exercises 23, 24, 25, 26, and 27 describe a situation. For each, identify all forces acting on the object and draw a free-body diagram of the object. Your physics textbook is sliding across the table.
543
views

 Verified step by step guidance
Verified step by step guidance