Magnesium citrate, Mg₃(C₆H₅O₇)₂, belongs to a class of laxatives called hyperosmotics, which cause rapid emptying of the bowel. When a concentrated solution of magnesium citrate is consumed, it passes through the intestines, drawing water and promoting diarrhea, usually within 6 hours. Calculate the osmotic pressure of a magnesium citrate laxative solution containing 28.5 g of magnesium citrate in 235 mL of solution at 37 °C (approximate body temperature). Assume complete dissociation of the ionic compound.

Question

Accepted Answer

Hello everyone today. We have been given the following problem and asked to solve for it Says assuming complete dissociation, calculate the osmotic pressure of magnesium acetate, abbreviated as such solution containing 52.1 g of magnesium citrate 352 ml of solution at 29°C. First you want to make note of the molar mass of magnesium citrate Which can be simplified to 142.4 g per mole. Next we want to take the amount of citrate that we actually have 52.1 g and transforms into moles by multiplying by the molar mass ratio. Our units are going to cancel and we're going to be left with 0. moles of Magnesium citrate. We now want to take our volume which is 352 ml and convert that into leaders. So we'll use the conversion factor That one mil leader is equal to 10 to the negative. 3rd leaders giving us 0.352 leaders. And now we can solve for malaria. So we take our polarity which is moles over L will take 0.366 moles And we'll divide that by 0.352 leaders to give us 1.04 m. And now we can use our osmotic osmotic pressure equation which is the osmotic pressure is equal to I or the Vantaa factor times polarity times r gas constant times our temperature. Now I is going to be three due to magnesium citrate dissociating into one magnesium and two citrate ions. So we have three overall Are malaria is 1.04. R. gas constant is 0.08-1 And our temperature is in C., but we want it in Kelvin, so we're just going to take the degrees in C and add 273.15 Kelvin. This gives us a final answer of 77. atmospheres. I hope this helped, and until next time.

Verified Solution

Key Concepts

Osmotic Pressure

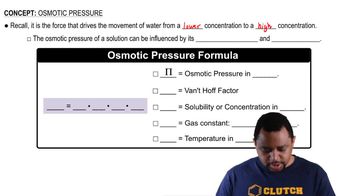
Van 't Hoff Factor

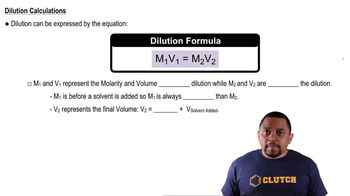
Molarity and Dilution