Textbook Question
Is it possible for a reaction to be nonspontaneous yet exo-thermic? Explain.
242
views
 McMurry 8th Edition
McMurry 8th Edition Ch.18 - Thermodynamics: Entropy, Free Energy & Equilibrium
Ch.18 - Thermodynamics: Entropy, Free Energy & Equilibrium Problem 128
Problem 128 Verified step by step guidance
Verified step by step guidance

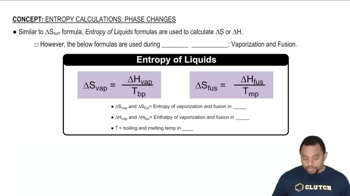

Trouton's rule says that the ratio of the molar heat of vaporization of a liquid to its normal boiling point (in kelvin) is approximately the same for all liquids: ∆Hvap/Tbp ≈ 88 J/(K*mol) (a) Check the reliability of Trouton's rule for the liquids listed in the following table.
Trouton's rule says that the ratio of the molar heat of vaporization of a liquid to its normal boiling point (in kelvin) is approximately the same for all liquids: ∆Hvap/Tbp ≈ 88 J/(K*mol) (b) Explain why liquids tend to have the same value of ∆Hvap/Tbp.