Match the equation in Column I with its solution(s) in Column II. x^2 + 5 = 0

Key Concepts

Quadratic Equations

A quadratic equation is a polynomial equation of the form ax^2 + bx + c = 0, where a, b, and c are constants, and a ≠ 0. The solutions to these equations can be found using various methods, including factoring, completing the square, or applying the quadratic formula. In this case, the equation x^2 + 5 = 0 is a specific type of quadratic equation.

Complex Numbers

Complex numbers are numbers that have a real part and an imaginary part, expressed in the form a + bi, where 'a' is the real part, 'b' is the imaginary part, and 'i' is the imaginary unit defined as the square root of -1. When solving the equation x^2 + 5 = 0, the solutions involve complex numbers since the equation does not have real solutions.

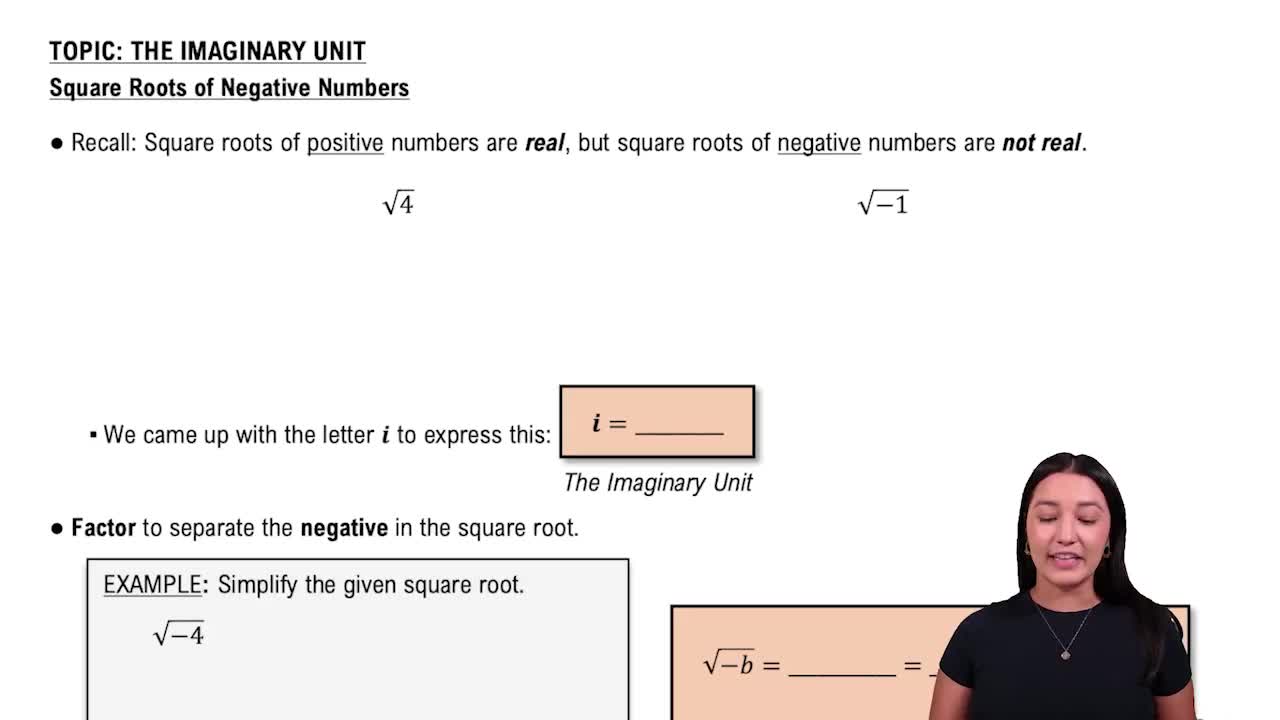
Square Roots of Negative Numbers

The square root of a negative number is not defined within the set of real numbers, leading to the introduction of imaginary numbers. For the equation x^2 + 5 = 0, we can rearrange it to x^2 = -5, which requires taking the square root of -5. This results in solutions expressed as x = ±√(-5) = ±i√5, illustrating the concept of square roots of negative numbers.