Here are the essential concepts you must grasp in order to answer the question correctly.
Distributive Property
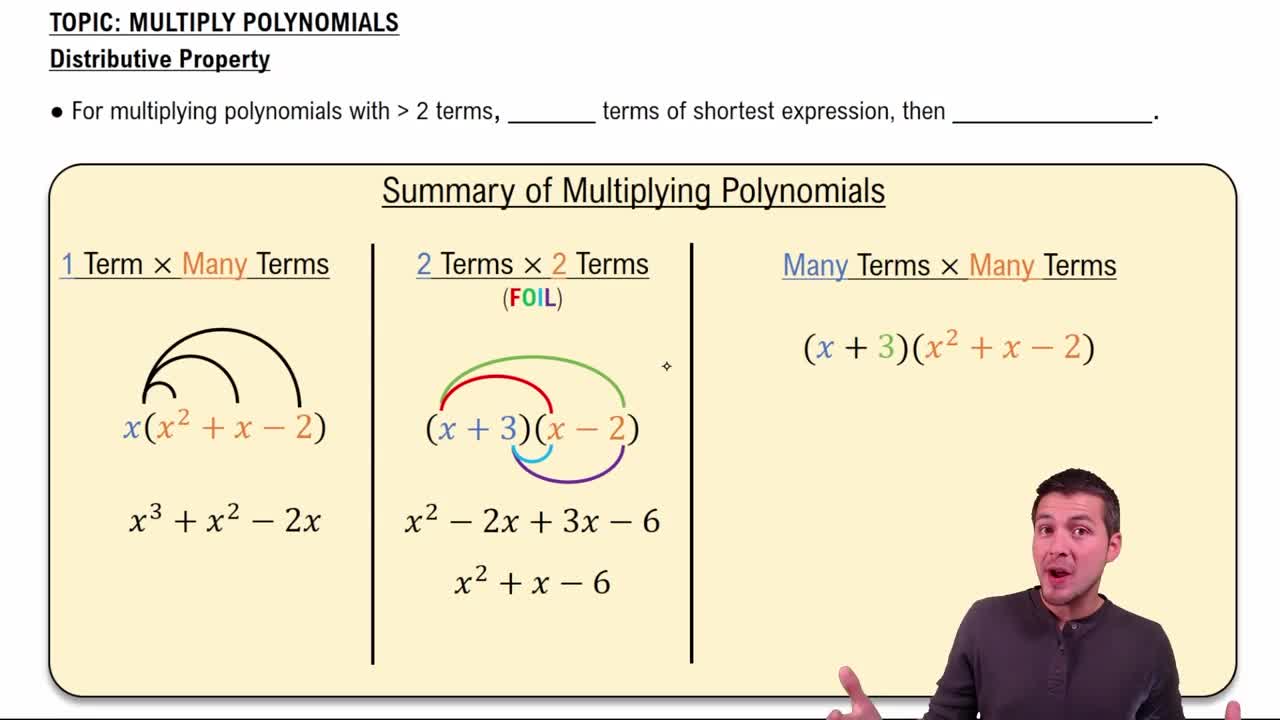
The Distributive Property states that a(b + c) = ab + ac. This property allows us to multiply a single term by each term inside a parenthesis. In the given equation, applying the distributive property is essential to simplify expressions like -2(x + 4) and 3(2x + 1) before combining like terms.
Recommended video:
Multiply Polynomials Using the Distributive Property
Combining Like Terms
Combining like terms involves adding or subtracting terms that have the same variable raised to the same power. This step is crucial in simplifying equations to isolate the variable. In the equation provided, after applying the distributive property, you will need to combine terms involving 'x' and constant terms to simplify the equation further.
Recommended video:
Solving Linear Equations
Solving linear equations involves finding the value of the variable that makes the equation true. This typically includes isolating the variable on one side of the equation through operations such as addition, subtraction, multiplication, or division. In the context of the given equation, the goal is to manipulate the equation until 'x' is isolated, revealing its value.
Recommended video:
Solving Linear Equations with Fractions