Match the equation in Column I with its solution(s) in Column II. x^2 - 5 = 0

Key Concepts

Quadratic Equations

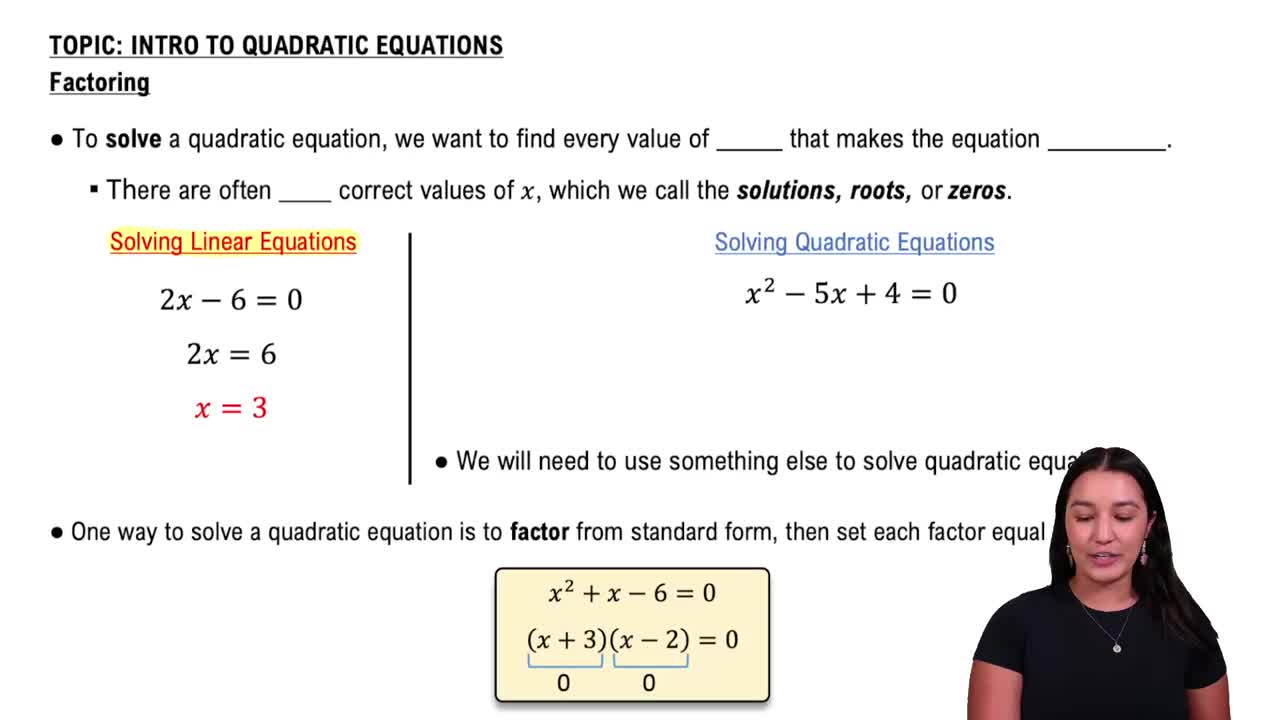
A quadratic equation is a polynomial equation of the form ax^2 + bx + c = 0, where a, b, and c are constants, and a ≠ 0. These equations can have zero, one, or two real solutions, depending on the discriminant (b^2 - 4ac). In this case, the equation x^2 - 5 = 0 is a simple quadratic equation.

Solving Quadratic Equations

To solve a quadratic equation, one can use various methods such as factoring, completing the square, or applying the quadratic formula: x = (-b ± √(b^2 - 4ac)) / (2a). For the equation x^2 - 5 = 0, we can isolate x^2 and take the square root to find the solutions, which will yield both positive and negative roots.

Real and Complex Solutions

Quadratic equations can yield real or complex solutions based on the value of the discriminant. If the discriminant is positive, there are two distinct real solutions; if it is zero, there is one real solution; and if it is negative, the solutions are complex. In the case of x^2 - 5 = 0, the solutions are real and can be expressed as x = ±√5.