Solubilty Product Constant - Online Tutor, Practice Problems & Exam Prep

Solubility indicates the maximum amount of solute that can dissolve in a solvent, forming a homogeneous mixture called a solution. The solubility product constant (Ksp) relates to ionic solids; a larger Ksp signifies greater solubility. The reaction quotient (q) helps determine if a reaction is at equilibrium by comparing it to Ksp. If q equals Ksp, the system is at equilibrium. If q is greater than Ksp, the reaction shifts toward reactants, increasing precipitate. Conversely, if q is less than Ksp, the reaction shifts toward products, enhancing solubility and ion production.

Solubility Product Constant

So here we're going to say that solubility represents the maximum amount of solute that could successfully dissolve in a solvent. Remember, when a solute successfully dissolves within a solvent, we create a homogeneous mixture known as a solution. Now we're going to say associated with any ionic solid is a Ksp value. This Ksp value is our solubility product constant. We're going to say here, the larger your solubility product constant, then the more soluble an ionic solid is in a solvent. So the more of it breaks up. And then we're going to say here the smaller the solubility product constant then the less soluble an ionic compound becomes within a solvent. So just remember, if we're looking at the solubility of any ionic compound, associated with it is a ksp value. Now that we've got that out of the way, click on to the next video and see how we look at example 1 when we're asked to figure out what our solubility product constant is for a hypothetical ionic compound when given its molar solubility.

Solubility Product Constant

So here we're told we have a hypothetical compound with the formula MX₃ and it has a molar solubility of 0.00562 molar. We're asked, what is the value of the solubility product constant for this? It's going to break up into its ions. The metal, which is M, breaks off. This little 3 here came from the metal, so its original charge was 3 plus. Remember, ions in solution are aqueous. Plus, there are 3 of the X negative ion. Here we have our equilibrium equation. With K_sp, we're talking about how it forms an equilibrium. And with equilibria, we'll set up an ICE chart. So we have initial, change, equilibrium. Remember, we ignore solids and liquids within an ICE chart. The whole thing is breaking up in pure water, so initially, our ions are 0 and 0. But their products are they're being formed, so the change is going to be plus x for this. This is a 3 here so this is plus 3. Bring down everything so plus x plus 3. Now, K_sp is an equilibrium constant and, like all other equilibrium constants, it equals products over reactants. But here when we're dealing with a saturated solution, we're going to say here that our ionic compound is a solid so it's going to be ignored. Remember, we ignore solids and liquids. So K_sp will just equal products. So in this case, it will equal M³⁺timesX⁻. The 3 here makes this 3 but it also cubes it. It make it becomes the power. We're going to plug in what we know at equilibrium for each one of these ions. So at equilibrium, M³⁺ is x and x⁻ is 3. So plug it in. So x times 3 cubed is 3 times 3 times 3, which is 27, and then this is x3. Multiply it together gives us 27 to the 4th. So that's what K_sp equals. Now realize here when they give us the solubility of the ionic compound, that solubility itself represents x. All we're going to do now is plug that value in for x. We'll write it over here. So K_sp equals 27 to the 4th. So that's 27 times 0.00562 which is gonna be to the 4th. What you're going to do here is take that number to the 4th, then multiply by 27. So 27 is gonna multiply 9.9754 times 10 to the negative 10. Multiply that by 27 so that comes out to 2.69 times 10 to the negative 8. And like other equilibrium constants, K_sp has no units. So it's just that number which gives us option D. Just realize here when they're telling us the molar solubility, they're giving us what x equals for the entire ionic compound. Using that is key to determining your K_sp. Now that you've seen example 1, take a look and see if you can do example 2. Here we're asked to figure out the highest molar solubility of each ionic compound given. Here we have to determine what the x variable will be. From our work in example 1, you just have to look at things backwards. Moving in the opposite direction will help us to isolate x for each situation. And from that, you'll be able to see which one has the highest x value and, therefore, the highest molar solubility.

Solubility Product Constant

Here it states, which of the following compounds will have the highest molar solubility in pure water? Now to determine which one has the smaller solubility, what we should do first is determine how many ions each ionic compound breaks up into. So first, we have cobalt(II) hydroxide. That'll break up into when we work it out, we're gonna have 1 cobalt(II) ion plus 2 hydroxide ions. That's 3 ions total that we have here. Next, we have strontium phosphate. That's going to break up into 3 strontium ions plus 2 phosphate ions. So, so far we have 3 ions here, 5 ions here. Next, we have lead(II) chloride. That breaks up into lead(II) ion plus 2 chloride ions. So that's 3 ions so far. Next, we have silver cyanide which breaks up into 1 silver ion plus 1 cyanide ion. So what do we have so far? Two ions there. And then finally, we have lead(II) sulfate which breaks up into lead(II) ion plus sulfate ion. Alright. So let's look at the ones that have number of ions in common. We have first, cobalt hydroxide and lead chloride. They both have the number of ions, so now we can look at their K_sp and determine which one is more, will have the higher molar solubility. Here, lead(II) chloride has a higher K_sp value because it's 10 to the negative 5. That means that it's more soluble than cobalt(II) hydroxide would be. Because it's more soluble, that means it's gonna have a larger molar solubility. So automatically, we know that c is greater than a. Next, we look at silver cyanide and lead sulfate because they both break up into 2 ions. Now comparing their K_sps here, we're going to say that this is to the negative 8 where this one is to the negative 17. So lead sulfate is more soluble. Therefore, it's going to break up more easily into its ions meaning you'll have a higher molar solubility. So this is out. What we have left are strontium phosphate, lead(II) chloride, and lead(II) sulfate. Here, we can't just simply look at them and look at their K_sps because they break up into a different number of ions. In this case, we'll have to determine what our molar solubility or x is for each ionic compound. We'll start out first with strontium phosphate and see how it's done. Alright. So we're gonna take this and work it out. So remember, we're dealing with an ionic solid breaking up. Since it's an ionic solid, this is out. These 2 are products that are being made so this is 0 and 0. There's a 3 here so this is plus 3 x. There's a 2 here so this is plus 2 x. Plus 3x, plus 2x. K_sp here will equal strontium ion and it's cubed because of the 3 as its coefficient, times phosphate ion squared because also its coefficient. So we're gonna say here that comes out to 3 x which is gonna be cubed, and 2 x which is gonna be squared. So that's 27 x to the 3rd times 4 x to the 2, which comes out to 108 x to the 5th. So my K_sp here, which is 4.0 times 10 to the negative 28 equals 108x to the 5th. Alright. So at this point, divide both sides here by 108. So when we do that, we're going to say now x to the 5th equals 3.70 times 10 to the negative 30. Take the 5th root of both sides, so x equals 1.30 times 10 to the negative 6 molar. This number here represents the molar solubility of the ionic compound itself. All we have to do now is do it also for lead(II) chloride and lead(II) sulfate. So we can get rid of this choice because we know that's not gonna be an answer. We're gonna bring this portion down We're here. Alright. So initial change equilibrium. X. So this is 0+0 plus x. There's a 2 here, so plus 2x, plus x, plus 2x. K_sp equals Pb²⁺ times Cl^- squared equals x times 2x squared, which comes out 4 x to the 3. And this equals 1.60 times 10 to the negative 5. Divide both sides here by 4. So that's gonna give me now x cubed equals 4.0 times 10 to the negative 6. Take the cube root of both sides now. And that's gonna give me x equals 0.0159 molar. So, so far we know that lead(II) chloride will be better than strontium phosphate because it has a larger x value. So its molar solubility is greater. Here this is initial change equilibrium again. 0. This is plus x and plus x, plus x and plus x. K_sp now equals Pb²⁺ times SO₄^2-. So that's x squared. K_sp is 1.82 times 10 to the negative 8. Take the square root of both sides here just to isolate x. So x here for the final one will equal 1.35 times 10 to the negative 4 molar. So we can see here that the answer has to be option c because it gave us the largest x value, so it has the largest molar solubility. So remember, for a question like this, the strategy is to first determine how many ions each break up into, then group the ionic compounds that break up into the same number of ions. When you do that, you compare their K_sps to one another. The one with the larger K_sp, in that case, would be more soluble and therefore have a higher molar solubility. Once you've done that, all you'll be left with is ionic compounds that each break up into different number of ions. In each case, you'll have to calculate what x is at the end. And by comparing, you'll know which one is the most soluble overall because it has the largest molar solubility.

Solubility Product Constant Calculations 1

Here, we're told that lanthanum(III) hydroxide has a K_sp of \(2.0 \times 10^{-21}\). It asks how many grams of lanthanum(III) hydroxide are dissolved as hydroxide ions in 2.5 liters of a saturated solution of lanthanum(III) hydroxide. Alright. So we're dealing with the K_sp of this compound. If we're dealing with K_sp, that means we're dealing with the solid form of this compound. And we look to see how it dissolves in water. When it dissolves in water, it establishes an equilibrium. So we're going to have one lanthanum(III) ion. Ions when dissolved in water are aqueous plus three hydroxide ions. Here, we're going to set up an ICE chart when dealing with K_sp. So we have initial, change, equilibrium. With an ICE chart, we ignore solids and liquids. So here, my solid will be ignored. Products are being formed over time, so this will be 0 plus x. Because this is a 3, this is plus 3x. Plus x plus x plus 3x. Now we're going to say K_sp, which is your solubility product constant, equals products overreactants just like all other equilibrium constants. But here for this equation, our reactant is a solid so we're going to ignore it. So K_sp here will just equal products. So it equals lanthanum(III) ion times OH^- because, again, this coefficient is 3. Now this is going to be to the third power. So that coefficient makes it \(3x\) but also makes it raised to that power. K_sp again is \(2.0 \times 10^{-21}\). At equilibrium, La³⁺ is x. OH^- is \(3x\). So that's x times \(3^3\) is 27, and then that's \(x^3\). So this comes out to \(27x^4 = 2.0 \times 10^{-21}\). So here, we need to isolate x here. Divide both sides here by 27. When we do that here, that's going to give me \(7.41 \times 10^{-23} = x^4\). Now we need to just isolate x, not \(x^4\). So I'm going to take the fourth root of both sides. So for some of you, you may see this button on your calculator. You'll see an x and then the square root symbol. What you would do is you'd punch in 4 first, then you hit that number. Brackets plug in this value that we have, close brackets and that'll give us x. For those of you who don't have that, you may have to hit, you would have to plug this number in still in parentheses. And you may see this button. You may see a caret button that points up like that, or you might see y to the x. So you'd have one of those 2 buttons, and then you do parenthesis \( \frac{1}{4} \) because the fourth root is the same thing as to the \( \frac{1}{4} \) power, and that would give you the same x as an answer. So that will give us \(x = 2.934 \times 10^{-6} \, \text{M}\). That is what our x variable is equal to. Anytime we find x, x here represents the solubility of my ionic compound.

If we go back to the original question, it's asking us to find grams. How many grams of lanthanum(III) hydroxide are dissolved as hydroxide ions? We're looking for grams of hydroxide ions. The wording is a little bit tricky, but that's what we're looking for. If we need to find the grams of hydroxide ions, we need to first figure out its concentration. At equilibrium, hydroxide is equal to \(3x\). So this \(x\) that we just found, plug it into here to find the concentration of OH^-. So again, OH^- equals \(8.80 \times 10^{-6} \, \text{M}\). Remember that moles equal liters times molarity. We're told that we have 2.5 liters. So multiply that by the molarity we just isolated for hydroxide ions and that'll give us the moles of hydroxide ions, which comes out to \(2.20 \times 10^{-5}\) moles of hydroxide ions. All we have to do now is just change those moles into grams. So one mole of hydroxide ions. We have 1 oxygen, 1 hydrogen. So looking at your periodic table, the masses are \(15.9994 \, \text{grams}\) and \(1.00794 \, \text{grams}\). Add them together gives us \(17.0073 \, \text{grams}\), roughly, of hydroxide ions. Moles cancel out, and we'll get grams at the end which comes out to \(3.74 \times 10^{-4} \, \text{grams}\) of hydroxide ion. So as you can see, there's a great deal of work that's required in terms of answering this question. Read the question very carefully. Here, we're not looking for grams of the entire ionic compound. We're looking for grams of lanthanum(III) hydroxide in the form of hydroxide ions. So we're actually looking for the grams of hydroxide ions in this question. Anytime we find x, x gives us the solubility or concentration of our ionic compound as a whole. If we want the concentration of the particular ions, we have to look at the ICE chart. At equilibrium, this ion which is just equal to x, so it'd be the same exact number. But for hydroxide ions, at equilibrium, it equals \(3x\). So it'd be 3 times what we found for x to get the concentration of hydroxide ion. From there, we can change concentration to moles by using liters and then change those moles into grams. Now that you've seen this example, move on ahead to example 2. See if you can approach this question. If you can't, don't worry. Just come back and see how I tackle this example 2 question.

Solubility Product Constant Calculations 1

Here it says find the pH of a saturated solution of aluminum hydroxide. We're told that the Ksp of aluminum hydroxide is 1.9 times 10 to the negative 10. We know that we're dealing with Ksp. We're dealing with this ionic solid and we're discussing how it breaks up into ions when thrown into solution. So it breaks up into aluminum ion plus 3 hydroxide ions. Hopefully, by following the example up above, you're able to get this far in terms of this problem. The steps are very similar to what we saw up above except now we're being asked to find pH. Here, we're gonna have initial change equilibrium. Again, remember solids and liquids are ignored within our ice chart. We're gonna say here, initially, this is 0 and this is 0. This is plus x. Just like up above, we have 3 OH^- here, so this is 3x. So plus x plus 3x. Ksp equals products over reactants, but we ignore the reactants because it's a solid. So it equals aluminum times OH^-. It's gonna be cubed because of the 3 as the coefficient. Ksp is 1.9 times 10 to the negative 10, x times 3x which will be cubed. So just like up above, we have 27x⁴ again equals 1.9 times 10 to the negative 10. Divide both sides here by 27, and we'll get what x to the 4th equals. So x to the 4th equals 7.03704 times 10 to the negative 12. Again, like up above, we're gonna take the 4th root, or you could do it to the 1/4^th power to find x. X here equals 1.629 times 10 to the minus 3 molar. This represents the concentration of my entire ionic compound. But remember, we're looking for pH here. Now in our equation, we don't have H₃O⁺ anywhere, but what we have instead is OH^-. If I know OH^-'s concentration, I can find my pOH. And from there, I can find pH. So let's figure out what the concentration of OH^- is. At equilibrium, OH^- equals 3x. So OH^- equals 3x. So that's 3 times 1.629 times 10 to the minus 3. So that's gonna come out to 4.886 times 10 to the minus 3 molar. Now that we have the molarity, we can figure out what my pH is. So first, we're gonna take the negative log of this number to find pOH which is the negative log of OH^-. So that comes out to 2.31. Now that we have pOH, we know pH because pH equals 14 minus pOH. That comes out to 11.69 as my pH for this solution. If you're able to follow along with the example up above, this question was similar up to a point. Once we found the concentration of OH^- in this example, we didn't need to figure out its grams or its moles. Instead, we'd use those concentrations that we found to figure out our pOH. From pOH, transform it into pH, and we'd have our answer conventions that we saw up above, we see that our pH is equal to 11.69.

Solubility Product Constant Calculations

So here it says, calculate the molar solubility of iron(II) carbonate in a solution of 0.00167 molar sodium carbonate. Here we're told that the K_sp for iron(II) carbonate is 2.1×10−11. Alright. We're dealing with iron(II) carbonate and we're given its K_sp, which means that we know we're dealing with the solid form of it. We look at how it breaks up into ions.

Now realize, up to this point, we're normally seeing these ionic compounds breaking up in pure water. But in this case, it's breaking up in a solution that is comprised of sodium carbonate. Realize sodium carbonate breaks up into sodium ion and carbonate ion. The carbonate ion is what's important because that carbonate ion is the same carbonate ion here. We're going to say here, because we're breaking up in a solution that already possesses carbonate ion, we're dealing with the common ion effect. With the common ion effect. This is a solid, so we're going to ignore it. There's no common ion of this. This is 0. With the common ion effect, because this carbonate which the solution is comprised of is the same as this ion here.

The initial concentration will be 0.00167. Then they're both products, so this is plus x, plus x, plus x and then 0.00167 plus x. Now with K_sp, remember we're concerned with the product because the reactant here is a solid. It's ignored. K_sp equals Fe²⁺ times CO₃²⁻. So here K_sp is 2.1×10−11 equals x times 0.00167. Now with K_sp, we're going to say because K_sp values are normally so low for the solubility of these ionic compounds, that means that we can ignore our x variable as long as there is a number that is in front of it that precedes it. So we're looking for the molar solubility of iron(II) carbonate, so that just means we're looking for x.

So divide both sides here by 0.00167. And when we do that, that's going to give us our x variable, which is our molar solubility for the entire ionic compound that comes out to 1.26×10−8 molar. Remember, with the common ion effect, we're no longer breaking up our ionic compound in pure water. We're breaking up in a solution that possesses a common ion to one of the ions in our ionic compound. Taking this to heart, attempt to do practice question 1. If you get stuck, don't worry. Just come back and take a look at how I approach that same exact practice question, and how we get the answer that we're looking for.

What is the molar solubility of Fe(OH) ₃ (s) in a solution that is buffered at pH 3.50 at 25°C? The Ksp of Fe(OH)₃ is 6.3 x 10^–38 at 25°C.

• A. 1.99x10^-27 M
• B. 1.99x10^-34 M
• C. 6.64x10^-28 M
• D. 1.99x10^-6 M
• E. 1.25x10^-9 M

Arsenic trisulfide (As₂S₃) occurs naturally as the orange-yellow colored mineral orpiment. As₂S₃ is a highly insoluble substance with a Ksp value of 2.90×10⁻⁷². Calculate the solubility of As₂S₃ in g/100mL. (Molar mass of As₂S₃ = 246.04 g/mol)

• A. 2.12×10⁻¹⁷ g/100 mL
• B. 1.87×10⁻¹³ g/100 mL
• C. 6.25×10⁻¹⁵ g/100 mL
• D. 4.75×10⁻¹⁴ g/100 mL

Reaction Quotient & Precipitation

So here we're talking about the reaction quotient q. We're going to say here that the reaction quotient q is used to determine if our chemical reaction is at equilibrium when we compare it to k or if we produce a precipitate solid. And that's when we compare it to ksp. Now remember, regular k is your equilibrium constant. Ksp is also an equilibrium constant but Ksp is an equilibrium constant for ionic solids.

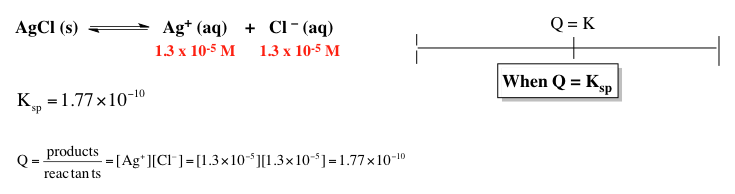
Now, here we're going to say if the reaction quotient q is equal to the equilibrium constant k, then our reaction is at equilibrium. Here, we're given the concentrations initially of silver and chloride ion as 1.3 × 10^-5 molar. Now, q is just like k. It equals products over reactants. And just like our equilibrium constant ksp, we ignore solids and liquids. So q equals just products so it equals silver ion times chloride ion.

Plugging those values in gives us our q value. Here, our q is 1.77 × 10^-10 just like ksp is 1.77 × 10^-10. They're equal to one another. We can say because q and k equal each other here, we're at equilibrium.

In terms of the shifting of our chemical reaction, there would be no shifting of our chemical reaction because our chemical reaction is at equilibrium. Now later on when we look up new values for q, if q happens to be different from k, this will determine the direction our chemical reaction will shift in order to reattain equilibrium conditions. This shifting can have an effect on the amount of reactant or product that's used or created.

Now click on to the next video and see what happens when our q value is different from our k value or ksp value.

Reaction Quotient & Precipitation

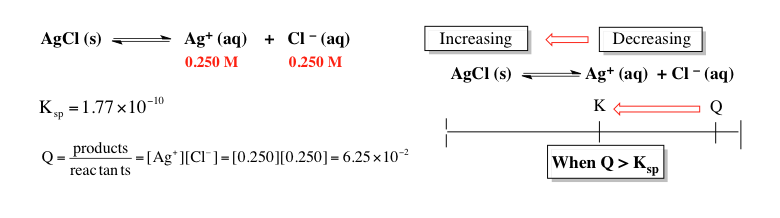
So here we continue our discussion of the comparison of the reaction quotient \( q \) to any type of equilibrium constant including \( K_{sp} \). Now in these next two scenarios, they will not be equal to one another. Because \( q \) and \( k \) will not be equal to one another, this will cause my chemical reaction to shift either in the forward direction or the reverse direction to reestablish equilibrium. Now in this first example here, we're given new concentrations for our ions. Here we have 0.250 molar for each one and when we plug them into the equilibrium expression for \( q \), we can see that the value of \( q \) comes out to \( 6.25 \times 10^{-2} \). In this case, we're going to see that \( q \) is bigger than our \( K_{sp} \) which is still the same value. Remember, the way this \( K_{sp} \) or any type of \( K \) would change is if there was a change in temperature. Our \( K_{sp} \) is remaining the same because temperature is being held constant but the concentration of my ions is changing which will have a direct impact on the value of \( q \). Here, \( q \) is larger than \( K_{sp} \). So if \( q \) is larger than \( K_{sp} \) or \( k \) in general, then our reaction will shift. So in terms of our number line, \( q \) will always shift to wherever \( k \) is. Here, \( q \) will shift this way to get to \( k \). If it shifts that way to get to \( k \), then our chemical reaction will also shift as a whole to get to equilibrium. Remember, wherever you shift, that direction will cause an increase. So here we're shifting towards the reactant side, so the reactant side would be increasing. If the reactant side is increasing, that means our product side is decreasing. We're going to say if \( q \) is larger than \( k \), then our chemical reaction will shift to the reactant side. In this case, because we're shifting towards the reactant which happens to be a solid, that means that the precipitate will increase. The amount of solid will increase.

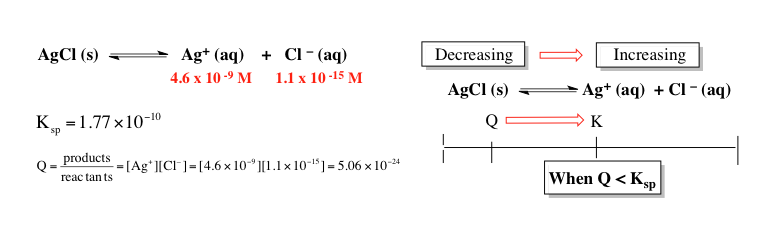
Now, here in this case, we have new concentrations yet again for the ions. Again, temperature is not being affected so our equilibrium constant \( K_{sp} \) remains the same. So in this case, we have these new concentrations which give us a new \( q \) value which is \( 5.06 \times 10^{-24} \). We can see now that that value is less than \( K_{sp} \). So if \( q \) is smaller than \( K_{sp} \) or \( k \) in general, so now \( q \) is no longer here. Instead, \( q \) will be over here. It's going to be smaller. So we're going to have to shift this way now. We're going to shift that way in terms of the number line and in terms of chemical reaction. Remember, wherever we're heading towards, that side is increasing. So this side would increase. This side here would decrease. Our chemical reaction will shift to the product side. That means that the solubility of my ionic compound is increasing because I'm making more ions and the amount of precipitator solid will decrease. Just remember, \( q \), our reaction quotient is just used as a way of determining are we at equilibrium. When \( q \) equals \( K_{sp} \), we're at equilibrium. We will say that our chemical reaction or actually our solution is a saturated solution with no real dominant movement to the reactant side of the product side. We're at equilibrium. Everything is balanced, so we'd represent a saturated solution. Then if our \( q \) happens to be larger than our \( K_{sp} \), that means we have an excess of ions so that's why the reaction is shifting in the reverse direction towards the reactants to recreate some more precipitate. We'd say that this type of process happens within a supersaturated solution. And then finally, when \( q \) is less than \( K_{sp} \), we can continue to break down our ionic solid to produce more ions. So when \( Q \) is less than \( K_{sp} \), we'd say that we are in an unsaturated solution. Now that we've explored the connection between our reaction quotient \( Q \) and \( K_{sp} \), we'll be more prepared when we take a look at questions dealing with more calculation-based questions on the formation or lack thereof of precipitates.

Reaction Quotient & Precipitation Calculations 1

Here it asks, will a precipitate form when 0.150 liters of 0.100 molar lead acetate and 0.100 liters of 0.20 molar sodium chloride are mixed. Here, we're told that the Ksp value of lead(II) chloride is 1.2×10-5. All right. We're given the Ksp of lead(II) chloride, so that's our ionic compound of interest. We're going to take that ionic compound and bring it down. So it's our solid and we show how it dissociates into its ions when it's thrown into our solution. That creates lead(II) ion, aqueous, plus 2 chloride ions. And here we have initial change equilibrium. Realize here at this point is, we have this lead(II) chloride forming and what's happening here is we have a solution made up of lead(II) acetate and sodium chloride. They're breaking up into ions and those ions are combining together to help form this solid here. All we're doing is showing its equilibrium equation. This lead(II) chloride exists in a solution made up of these 4 particular ions. It's not being formed in pure water. And if you look, those 4 ions from those compounds, we have lead(II) ion in it and we care about that because that's part of my equilibrium equation. And then we have chloride ion here which is also part of my equation. Here we're dealing with double common ion effect. These amounts that we're going to find for both the lead(II) ion and the chloride ion will be used to help us determine what Q will be.

We have to figure out what the concentration of this lead(II) ion is and the concentration of this chloride ion is. Remember, liters of a molarity, of means multiply. Remember that moles equals liters times molarity. So we take 0.150 liters, multiply it by the 0.100 molar of lead(II) acetate. That's gonna give me 0.0150 moles of lead(II) acetate. Realize here that we don't want the moles of lead(II) acetate. We just want the moles of lead(II) ion because that is one of our common ions. We're going to say here for every one mole of the entire compound, we have exactly 1 mole of lead(II) ion involved. Now, we need to determine its concentration or its molarity. Realize here that we have 0.150 liters of one compound mixing with exactly 0.100 liters of another compound. Their combined volume forms the new overall volume of the solution. For the moles we just found, we're going to divide it by the total volume. Doing that will give me my new concentration for lead(II) ions which comes out to 0.060 molar. Now we have to do the same thing and figure out the new concentration of chloride ions. So we have 0.100 liters of 0.20 molar sodium chloride. Again, remember that liters times molarity will give me moles. So bring that down. We don't want the moles of sodium chloride. We just want the moles of chloride ion itself. So for every 1 mole of sodium chloride, we can see that there's exactly 1 chloride in the formula. So that's 0.020 moles of chloride ions. Again, we wanna find its new concentration, Together, all of that gives me a molarity of 0.080 molar for my chloride ions.

So take that concentration and plug it in. So now we have initial concentrations for both. So this would be plus x plus 2x, 0.060 plus x, 0.080, plus 2x. Because we have initial concentrations for both ions, this is going to help me figure out what my Q value will be. So we're going to say here Q=PV2+×Cl-2. So it's gonna be 0.060 times 0.080 squared. When we plug that in, that gives me 3.84-4 as my Q value. Now remember, what we said in the previous videos is at this point, when we find Q, we can compare it to our Ksp using a number line. That'll dictate which direction my chemical reaction will shift. Here's our number line here. We'll put Ksp in the middle. So we're told that Ksp of lead(II) chloride is 1.2×10-5. We can see that Q is this number, 3.84 times 10 to the negative 4. Here, it's to the negative 4, so it's a larger value. So Q will be over here. All we say now at this point is because Ksp and Q are not equal to each other where you are not at equilibrium, Q will shift in the direction needed to get to Ksp. So Q will shift this way to get to KSP. Remember, whichever direction you shift in to get to K is the same direction your reaction will shift to as well. Here, my chemical reaction would have to shift also this way. Wherever you're shifting will be increasing in amount. So my reactant here is increasing because we're shifting towards the reactants and this side here is decreasing. We're saying here that my precipitate, which is my solid, is increasing in amount. We would say yes, a precipitate does form. Remember, when Q is greater than Ksp, a precipitate will begin to form because our solution represents a supersaturated solution. And to get back to equilibrium, some of the extra dissolved solute has to recrystallize in the solution itself. Again, here because Q is greater than Ksp, our reaction moves in the reverse direction towards reactants, thereby creating more solid. Now that you've seen this example, look to see if you can tackle example 2. If you get stuck or lost, don't worry. Just come back and take a look at how we approach example 2.

Reaction Quotient & Precipitation Calculations 1

So here the question states, what is the minimum pH at which iron(II) hydroxide will precipitate if the solution has a concentration of iron(II) ion equal to 0.0583 molar. Here we're told that the K_sp of iron(II) hydroxide is 4.87 times 10^-17. This question here, although it's asking for precipitation, is really piggybacking off of the idea of just the simple common ion effect of iron(II) ions. We're going to write the ionic compound. We know here it's going to form an equilibrium with its ions: Fe²⁺ + 2 hydroxide ions.

We will have initial change equilibrium. We ignore the solid. Here, this iron(II) hydroxide compound is not breaking up in pure water, but in fact, the solution already comprises iron ions. We have 0.0583 molar iron ion. That there represents the initial concentration. Here, we're going to say the initial concentration for hydroxide is 0 since we don't have that common ion at all present in our solution. So this is going to be + x + 2x 0.0583 + x + 2x. Here, we're dealing with K_sp. We're not dealing with Q; we dealt with Q in the previous question because we had initial amounts for both of our products initially. Here, since we only have one of them, it's just a simple common ion effect that also encompasses a question dealing with precipitation.

So here, K_sp equals Fe²⁺ times OH^- will be squared equals 4.87 × 10^-17. Remember, when we have an actual value, we can ignore the variable because the variable is going to be such a small number it's not going to have a heavy impact on the value we have here. That's going to be 0.0583 times 2x which is going to be squared. Alright, so here, we're going to have 0.0583. so 2x squared. So 2 becomes squared, so that's going to become 4, and that's x squared.

Then here we're going to have this times 4, which is going to give me 0.2332 times x squared. Divide both sides by 0.2332. So x squared is going to equal 2.08834 × 10^-16. We want the square root of both sides now. So x here at this point equals 1.445 × 10^-8 molar.

Now they're asking us for the minimum pH. Realize here that pH is just the negative log of the H⁺ concentration. What we have that's similar to that is OH^-. So what we're going to do here is we're actually going to figure out what the concentration of OH^- is first. So, at equilibrium, OH^- equals 2x. So plug in the x that we just found. So that's going to give me a concentration of 2.89 × 10^-8 molar as the concentration of my hydroxide ion. If we know 2.89 × 10^-8. So that's going to give me 7.54. So that's my pOH. I don't want pOH. I want pH. pH equals 14 minus pOH, so it's 14 minus 7.54, which comes out to 6.46. That there represents the minimum pH at which a precipitate of iron(II) hydroxide can become a precipitate. Now realize here again we didn't use Q in this question because we didn't have the initial concentrations.