So when we're dealing with the F-test, remember the F-test is used to test the variance of 2 populations, and remember that variance is just your standard deviation squared. Now we're going to say Fcalculated represents the quotient of the squares of the standard deviations. Here it is standard deviation 1 squared divided by standard deviation 2 squared. We're going to say when calculating our F quotient, so basically this value, always set the largest standard deviation as the numerator, so that way Fcalculated will always be equal to or greater than 1. Now, we're going to say here we can compare our Fcalculated value to our F table value to determine if there is a significant difference based on the variances. Here we're going to say if your Fcalculated is less than your F table, then the difference will not be significant. So here we'd say that they would have equal variances, and as a result, our tcalculated and Spooled formulas would be these 2 here. Here x one is just the measurements, the mean or average of your first measurements minus the mean or average of your second measurements divided by Spooled, n is just the number of measurements. So population 1 has this set of measurements, population 2 has its own set of measurements. Here if your Fcalculated is greater than your F table, then there is a significant difference. So we're going to say here you'd have unequal variances, which would mean that you'd use a different set of values. Here this would be the equation to figure out tcalculated and then this would be our formula to figure out your degrees of freedom. Remember we've seen these equations before in our exploration of the T-test. And here is our F table. So your degrees of freedom for standard deviation 1, which is the largest standard deviation, and these are your degrees of freedom for standard deviation 2. Just like with the T table, you just have to look to see where the values line up in order to figure out what your T table value would be and then compare it to your F table value would be and then compare it to your Fcalculated value. As we explore deeper and deeper into the F-test, you'll see how we use this particular chart with questions dealing with the F-test.
- 1. Chemical Measurements1h 50m
- 2. Tools of the Trade1h 17m
- 3. Experimental Error1h 52m
- 4 & 5. Statistics, Quality Assurance and Calibration Methods1h 57m
- 6. Chemical Equilibrium3h 41m
- 7. Activity and the Systematic Treatment of Equilibrium1h 0m
- 8. Monoprotic Acid-Base Equilibria1h 53m
- 9. Polyprotic Acid-Base Equilibria2h 17m
- 10. Acid-Base Titrations2h 37m
- 11. EDTA Titrations1h 34m
- 12. Advanced Topics in Equilibrium1h 16m
- 13. Fundamentals of Electrochemistry2h 19m
- 14. Electrodes and Potentiometry41m
- 15. Redox Titrations1h 14m
- 16. Electroanalytical Techniques57m
- 17. Fundamentals of Spectrophotometry50m
Analysis of Variance (f-Test) - Online Tutor, Practice Problems & Exam Prep
 Created using AI
Created using AIThe F-test is utilized to compare the variances of two populations, calculated as the ratio of their squared standard deviations. Always place the larger standard deviation in the numerator to ensure the F-calculated value is ≥ 1. If F-calculated < F-table, variances are equal; if F-calculated > F-table, variances are unequal. This affects the formulas for t-calculated and degrees of freedom, which are essential for hypothesis testing in statistics.
An F-Test is used to compare 2 populations' variances.
F-Test
F-Test
Video transcript
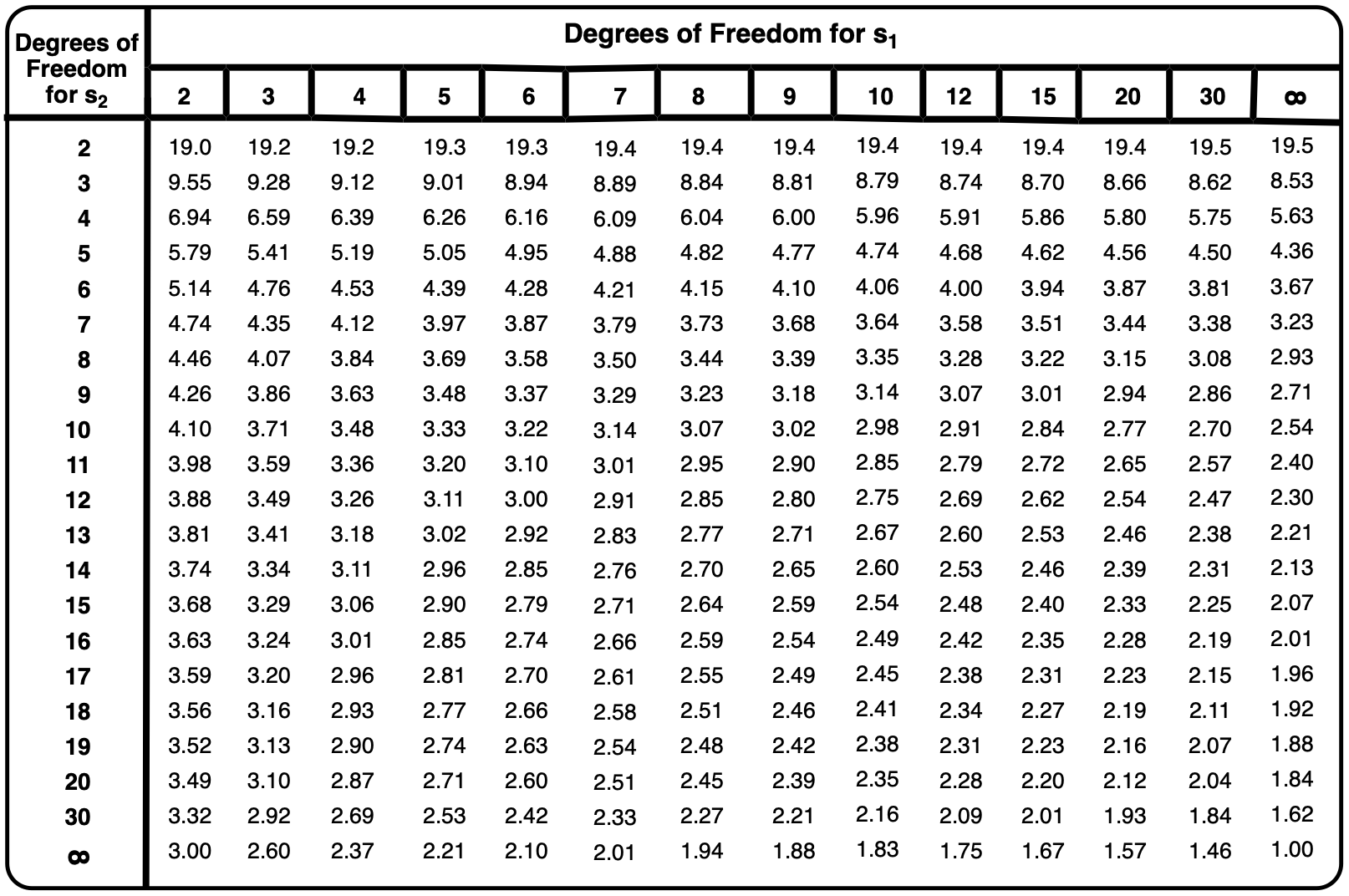
F-Test Calculations
F-Test Calculations 1
Video transcript
In the process of assessing responsibility for an oil spill, two possible suspects are identified. To differentiate between the two samples of oil, the ratio of the concentration for two polyaromatic hydrocarbons is measured using fluorescent spectroscopy. These values are then compared to the sample obtained from a body of water. So, we have information on our suspects and the sample we're testing them against. As a result, we have the averages or mean, the standard deviations of each, and the number of samples of each.
Here we're asked if any combination of the standard deviations demonstrates a significant difference. To determine this, we need to calculate the F-value for each combination. If the F-calculated happens to be greater than our F-table value, then there would be a significant difference. Firstly, we need to determine our degrees of freedom for each standard deviation, calculated as \( n - 1 \). This gives us 3, 4, and 5 respectively.
To calculate our F-values, we use the formula: F = s 1 2 s 2 2 , where \( s_1 \) is the larger standard deviation.
After comparing different combinations of standard deviations, the calculated F-values are:
- For \( s1 \) and \( s2 \): \( F \) equals 1.58829
- For \( s1 \) and \( s2 \): \( F \) equals 1.45318
- For \( s1 \) over \( s2 \): \( F \) equals 1.09298
We then compare these F-calculated values to the F-table values, which depend on the degrees of freedom associated with each standard deviation. For all combinations:
- Combination 1 - \( F_{table} \) is 9.12
- Combination 2 - \( F_{table} \) is 9.01
- Combination 3 - \( F_{table} \) is 5.19
Since there is no significant difference, this will influence how we proceed with subsequent analyses, as highlighted in example 2. If uncertain about the next steps, refer to further instructional material or videos for guidance.
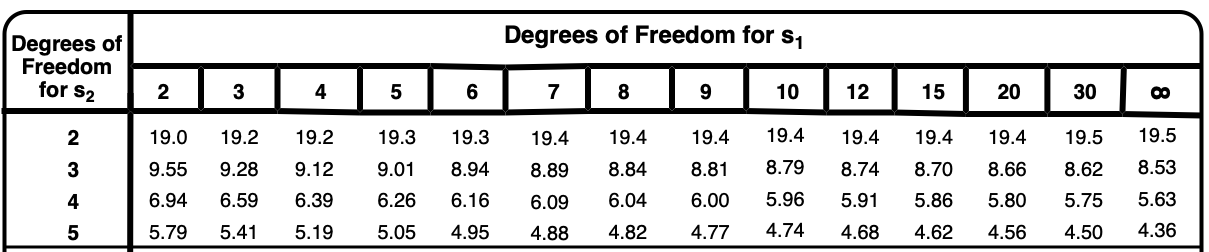
F-Test Calculations 1
Video transcript
So in example 2, it states, can either or both of these suspects be eliminated based on the results of the analysis at the 99% confidence interval? Now realize here because in example 1 we found out there was no significant difference in their standard deviations, that means we're dealing with equal variance. Because we're dealing with equal variance, this dictates what version of pooled standard deviation and calculated t formulas we'll have to use.
Now since there's going to be a lot of numbers on the screen, I'll have to take myself out of the image for a few minutes. So let's look at suspect 1 and then we'll look at suspect 2, and we'll see if either one can be eliminated.
For suspect 1, again, we're dealing with equal variance in both cases, so therefore s pooled = s1 × (n1 - 1) + s2 × (n2 - 1) / (n1 + n2 - 2) . So for suspect 1, using the values from these two for suspect 1. So we'll come back down here and before we come back actually, we're going to say here because the sample itself. So we'll make this one s1 and we can make this one s2, but it really doesn't matter in the grand scheme of our calculations.
So we come back down here. We'll plug in as s 1 2 = 1.073 ² × (4 - 1) + s 2 2 = 0.088 ² × (6 - 1) divided by 4 + 6 - 2. When we plug all that in, that gives a square root of 0.006838. And that comes out to 0.082694 for spooled.
Now that we have spooled we can figure out what t calculated would be. So t calculated because we have equal variance equals in absolute terms x₁ average x₁ minus x₂ divided by spooled times n1 times n2 over n1+n2. So the mean or average for suspect one is 2.31, and for the sample 2.45. We've just found out what spooled was, so plug that in. Times the number of measurements, so that's 4 times 6 divided by 4 +6. So all of that gives us 2.62277 for our t calculated.
Now if t calculated is larger than t table, then there would be a significant difference between the suspect and the sample. Here, if you use a t table, our degrees of freedom are normally n minus 1, but when it comes to comparing the two to one another, my degrees of freedom now become n1 plus n2 minus 2. So that would be 4 plus 6 minus 2, which gives me a degree of freedom of 8, 8. So if you go to your t table, look at 8 for the degrees of freedom, and then go all the way to the 99% confidence interval, that gives us a t table value equal to 3.355. So what is this telling us? Well, what this is telling us, it's telling us that our t calculated is not greater than our t table. T table is larger. T table is this, and t calculated is only this. So that means there is no significant difference between suspect 1 and the sample. So that will mean that suspect 1 is guilty of the oil spill because t calculated is less than t table, there's no significant difference. So suspect 1 is a potential violator.
Now let's look at suspect 2. So suspect 2, we're going to do the same thing, s pooled = s1 × (n1 - 1) + s2 × (n2 - 1) / (n1 + n2 - 2) , but now we're using different values. We're comparing suspect 2 now to the sample itself. So suspect 2 has a standard deviation of 0.092 which will square, times its number of measurements which is 5 minus 1, plus the standard deviation of the sample, and that's also squared. It had 6 samples, minus 1, divided by 5 + 6 - 2. So here that gives us square root of 0.008064. So that equals 0.0898. Now that we have spooled we can find t calculated here, which would be the same exact formula we used here. So here the mean of my suspect 2 is 2.67 minus 2.45 divided by my spooled, which we just found, times 5 times 6 divided by 5 + 6. So that's 2.44989 times 1.65145. So t calculated here equals 4.04586.
My degrees of freedom would be 5 + 6 - 2 which is 9, so we look up 9 for our degrees of freedom. We go all the way to the 99 confidence interval. So t table equals 3.250. So in this example, t calculated is greater than t table. So that means there is a significant difference between the sample and suspect 2, which means that they are innocent.
F-Test Calculations 2
Video transcript
Here it says you are measuring the effects of a toxic compound on an enzyme. You expose 5 test tubes of cells to 100 microliters of a 5 part per million aqueous solution of the toxic compound and mark them as treated. And expose 5 test tubes of cells to an equal volume of only water and mark them as untreated. You then measure the enzyme activity of cells in each test tube. Enzyme activity in this case is in units of micromoles per minute. Here are the following other measurements of enzyme activity. We're given here 2 columns. We have our enzyme activity that's been treated and enzyme activity that's been untreated. We have 5 measurements for each one. From this, we're able to obtain our average or mean for each one. We're also given our standard deviation. Now this question says, is the variance of the measured enzyme activity of cells exposed to the toxic compound equal to that of cells exposed to water alone?
Alright, so we know that variance, if we're trying to compare the variance between 2 samples or 2 sets of samples, that means we're relying on the F test. What we have to do here is we have to determine what the F calculated value will be. Remember, F calculated equals s1²÷s2². s1, the one on top is always the larger standard deviation, so that means that our F calculated at the end must always be a value that is equal to or greater than 1.
So here are our standard deviations for the treated and untreated. So we always put the larger standard deviation on top again. 0.362÷0.292. When we do that, it's going to give me 1.54102 as my F calculated. What I do now is remember on the previous page where we're dealing with F tables, we have 5 measurements for both, treated untreated. And if we line them up perfectly that means our F table would be 5 0.05. All we do now is compare our F table value to our F calculated value. So here, F calculated is 1.54102. F table is 5.05. If F table is greater than F calculated, that means we're going to have equal variance. So that just means that there is not a significant a significant difference. So since there's not a significant difference, this will play a major role in what we do in example 2. So work this example 2 out if you remember when your variances are equal, what set of formulas do we use. If you still can't quite remember how to do it or how to approach it, just click on to the next video and see how I answer example 2.
F-Test Calculations 2
Video transcript
Alright guys, because we had equal variance in example 1, that tells us which series of equations to use to answer example 2. So here it says is the average enzyme activity measured for cells exposed to the toxic compound significantly different at 95% confidence level, than that can be measured for cells exposed to water alone. Because we're going to have to utilize a few equations, I'm going to have to take myself out of the image guys but follow along. Again, we had equal variance according to example one. That tells me that I have to use t calculated. And we're going to use the version that is equal to absolute value of average 1 minus average 2 divided by spooled times square root of N1 N2 divided by N1 + N2.
And then here because we need spooled, spooled in this case would equal square root of standard deviation 1 squared times the number of measurements minus 1 plus standard deviation 2 squared, number of measurements minus 1 divided by N1 + N2 minus 2.
Now, we're used to seeing the degrees of freedom as being n - 1, but because here we're using 2 sets of data, our new degrees of freedom actually becomes N1 + N2 - 2. All right. Now all we have to do is plug in the values to get our t calculated.
So let's first figure out what our spooled will be. So it equals. So up above, we said that our standard deviation 1, which is the largest standard deviation, is 0.36. That'll be squared. The number of measurements is 5 minus 1, plus smaller deviation is 0.29 squared, 5 minus 1, divided by 5+5 minus 2. So when we figure out everything inside, that gives me square root of 0.10685. Taking the square root of that gives me an spooled equal to 0.32679.
So that's my spooled. So that's going to go here in my formula. So here T calculated equals 3.84 minus 6.15, from up above in absolute terms, divided by spooled, which we calculated as 0.32679, times 5 times 5 divided by 5 plus 5. So that gives me 7.06684 times 1.58114. Multiplying them together, I get a t calculated that is 11.1737.
Now we have to determine if they're significantly different at a 95% confidence level. So here, we need to figure out what our ttable is. So if you take out your t tables, we'd say that our degrees of freedom, remember, our degrees of freedom would normally be n - 1. But when dealing with the f test here, the degrees of freedom actually become this, N1 + N2 - 2. So that's 5+5 minus 2, so that's going to be a degree of freedom of 8. And we look at a degree of freedom of 8. We look at the 95% confidence interval, so my ttabled value equals 2.306.
So now we compare ttable to tcalculated. So we're going to say here that t calculated is 11.1737 which is greater than ttable, which is 2.306. Because of this, because t calculated is greater than ttable, that means we have to reject the hypothesis as being significantly different. So we're going to say, yes, significantly different between the 2 based on a 95% confidence interval or confidence level.
