Here are the essential concepts you must grasp in order to answer the question correctly.
Buoyancy
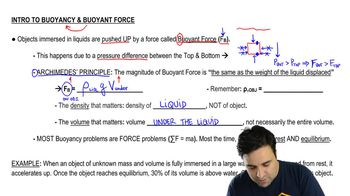
Buoyancy is the upward force exerted by a fluid on an object submerged in it. This force is equal to the weight of the fluid displaced by the object, as described by Archimedes' principle. In this scenario, the wooden block's buoyancy allows it to float at the interface between oil and water, with the extent of submersion determined by the densities of the fluids involved.
Recommended video:
Intro to Buoyancy & Buoyant Force
Gauge Pressure
Gauge pressure is the pressure relative to atmospheric pressure, measured in a fluid at a specific depth. It can be calculated using the formula P = ρgh, where ρ is the fluid density, g is the acceleration due to gravity, and h is the depth of the fluid above the point of measurement. In this problem, gauge pressure is relevant for determining the pressures at the upper and lower faces of the block.
Recommended video:
Pressure Gauges: Barometer
Density
Density is defined as mass per unit volume and is a key property of materials that influences their behavior in fluids. The density of the wooden block, along with the densities of oil and water, determines how much of the block is submerged and its buoyancy. Understanding the relationship between mass, volume, and density is essential for solving the problem regarding the block's mass and density.
Recommended video:

 Verified step by step guidance
Verified step by step guidance