Here are the essential concepts you must grasp in order to answer the question correctly.
Buoyancy
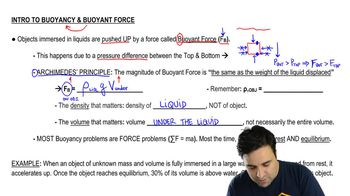
Buoyancy is the upward force exerted by a fluid on an object submerged in it. This force is equal to the weight of the fluid displaced by the object, as described by Archimedes' principle. In this scenario, the buoy will float until the weight of the man and the buoy equals the weight of the water displaced, determining how much it sinks.
Recommended video:
Intro to Buoyancy & Buoyant Force
Weight and Density
Weight is the force exerted by gravity on an object, calculated as the product of mass and gravitational acceleration (W = mg). Density, defined as mass per unit volume, plays a crucial role in determining whether an object will float or sink. The combined weight of the buoy and the man must be compared to the weight of the displaced seawater to find the additional sinking distance.
Recommended video:
Volume Displacement
Volume displacement refers to the volume of fluid that is moved out of the way when an object is submerged. The additional distance the buoy sinks can be calculated by determining the volume of water displaced by the added weight of the man. This volume can then be converted into a height by considering the cross-sectional area of the buoy.
Recommended video: