A remote-controlled car is moving in a vacant parking lot. The velocity of the car as a function of time is given by v = [5.00 m/s − (0.0180 m/s3)t2]î + [2.00 m/s + (0.550 m/s2)t]ĵ. (a) What are ax(t) and ay(t), the x- and y-components of the car's velocity as functions of time?

Verified step by step guidance

1. Identify the given velocity components of the car. The x-component of the velocity is given by vx(t) = 5.00 m/s - (0.0180 m/s^3)t^2 and the y-component of the velocity is vy(t) = 2.00 m/s + (0.550 m/s^2)t.
2. Recall that acceleration is the derivative of velocity with respect to time. Therefore, to find the x-component of acceleration, ax(t), differentiate the x-component of velocity, vx(t), with respect to time t.
3. Differentiate vx(t) = 5.00 m/s - (0.0180 m/s^3)t^2 with respect to t to find ax(t). Use the power rule for differentiation, where the derivative of at^n with respect to t is nat^(n-1).
4. Similarly, to find the y-component of acceleration, ay(t), differentiate the y-component of velocity, vy(t), with respect to time t.
5. Differentiate vy(t) = 2.00 m/s + (0.550 m/s^2)t with respect to t to find ay(t). Again, use the power rule for differentiation.

Key Concepts

Velocity as a Function of Time

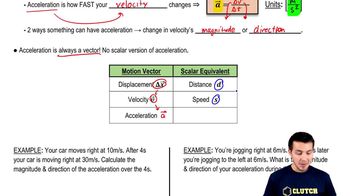
Velocity is a vector quantity that describes the rate of change of an object's position with respect to time. In this case, the velocity of the remote-controlled car is expressed as a function of time, indicating that both the x- and y-components of the velocity change over time. Understanding how to interpret and manipulate these functions is crucial for determining the car's motion.

Components of Velocity

Velocity can be broken down into its components along the x and y axes, represented as vx(t) and vy(t). This decomposition allows for easier analysis of motion in two dimensions. In the given equation, the x-component and y-component of the velocity are defined separately, which is essential for calculating the acceleration and other related quantities.

Differentiation and Acceleration

Acceleration is defined as the rate of change of velocity with respect to time. To find the acceleration components ax(t) and ay(t), one must differentiate the velocity components vx(t) and vy(t) with respect to time. This process is fundamental in physics, as it connects the concepts of motion and forces acting on an object.