Here are the essential concepts you must grasp in order to answer the question correctly.
Frequency and Beats
Frequency refers to the number of cycles of a wave that occur in a unit of time, measured in Hertz (Hz). When two sound waves of slightly different frequencies interact, they produce a phenomenon known as beats, which is the periodic variation in amplitude resulting from the interference of the two waves. The beat frequency is equal to the absolute difference between the two frequencies.
Recommended video:
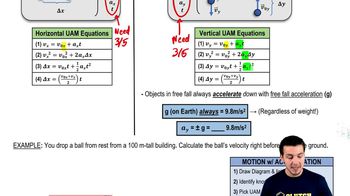
Free Fall and Acceleration
Free fall describes the motion of an object under the influence of gravity alone, with an acceleration of approximately 9.81 m/s² near the Earth's surface. In this scenario, the box dropped from the balcony will accelerate downward until it hits the ground, and the time it takes to fall can be calculated using the kinematic equations of motion.
Recommended video:
Vertical Motion & Free Fall
Time of Flight Calculation
The time of flight for an object in free fall can be determined using the equation t = √(2h/g), where h is the height from which it is dropped and g is the acceleration due to gravity. This calculation allows us to find out how long the box takes to reach the ground, which is essential for determining how many beats will be heard during that time.
Recommended video:
Calculating Displacement from Velocity-Time Graphs