Here are the essential concepts you must grasp in order to answer the question correctly.
Moment of Inertia
Moment of inertia is a measure of an object's resistance to changes in its rotational motion. It depends on the mass distribution relative to the axis of rotation. For a solid object, it can be calculated using specific formulas that take into account the shape and mass of the object, as well as the distance from the axis of rotation.
Recommended video:
Intro to Moment of Inertia
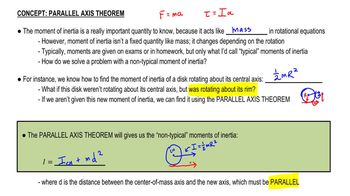
Parallel Axis Theorem
The parallel axis theorem allows us to calculate the moment of inertia of an object about any axis parallel to an axis through its center of mass. It states that the moment of inertia about the new axis is equal to the moment of inertia about the center of mass axis plus the product of the mass and the square of the distance between the two axes. This theorem is essential for solving problems involving rotation about axes that do not pass through the center of mass.
Recommended video:
Geometry of the Door
Understanding the geometry of the door is crucial for calculating its moment of inertia. The door can be approximated as a rectangular shape, and its dimensions (height and width) will influence the distribution of mass. Knowing the dimensions allows for the application of the appropriate formulas to determine the moment of inertia about the specified axis, taking into account the distance from the edge.
Recommended video:
Angular Momentum of Objects in Linear Motion
 Verified step by step guidance
Verified step by step guidance