Here are the essential concepts you must grasp in order to answer the question correctly.
Newton's First Law of Motion
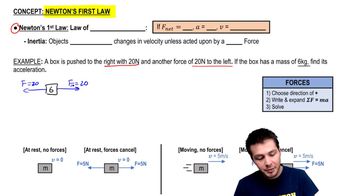
Newton's First Law states that an object at rest will remain at rest, and an object in motion will continue in motion at a constant velocity unless acted upon by a net external force. In this scenario, for the box to slide down at a constant speed, the net force acting on it must be zero, meaning the applied force must balance the forces of gravity and friction.
Recommended video:
Friction
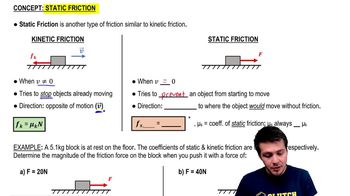
Friction is the resistive force that opposes the motion of an object sliding over a surface. It depends on the nature of the surfaces in contact and the normal force acting between them. In this case, the frictional force will play a crucial role in determining the magnitude of the force needed to keep the box moving at a constant speed down the wall.
Recommended video:
Static Friction & Equilibrium
Components of Forces
When a force is applied at an angle, it can be resolved into horizontal and vertical components using trigonometric functions. For the 45° angle in this problem, both the horizontal and vertical components of the applied force will affect the net force acting on the box. Understanding how to decompose forces is essential for calculating the required force to achieve constant speed.
Recommended video:
Vector Addition By Components