Here are the essential concepts you must grasp in order to answer the question correctly.
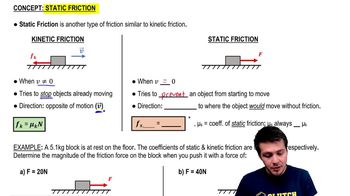
Friction
Friction is the force that opposes the relative motion of two surfaces in contact. It is characterized by two coefficients: static friction (μₛ), which prevents motion, and kinetic friction (μₖ), which acts when surfaces are sliding against each other. Understanding these coefficients is crucial for determining the conditions under which the small box will remain stationary on the large box as it decelerates.
Recommended video:
Static Friction & Equilibrium
Newton's Second Law
Newton's Second Law states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass (F = ma). This principle is essential for analyzing the forces acting on both boxes during the deceleration of the large box, allowing us to calculate the necessary forces to prevent slipping.
Recommended video:
Intro to Forces & Newton's Second Law
Kinematics of Uniformly Accelerated Motion
Kinematics describes the motion of objects without considering the forces that cause the motion. In this scenario, we need to apply the equations of motion for uniformly accelerated motion to find the shortest stopping distance (dₘᵢₙ) of the large box. This involves using the initial speed, final speed, and acceleration to derive the distance over which the box can stop without the small box slipping.
Recommended video: