Here are the essential concepts you must grasp in order to answer the question correctly.
Friction
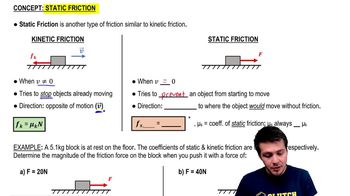
Friction is the force that opposes the relative motion of two surfaces in contact. It is characterized by two coefficients: static friction (μₛ), which prevents motion, and kinetic friction (μₖ), which acts when surfaces are sliding against each other. In this scenario, the static friction will determine whether the book can remain at rest at the highest point after being pushed up the slope.
Recommended video:
Static Friction & Equilibrium
Kinematics
Kinematics is the branch of mechanics that deals with the motion of objects without considering the forces that cause the motion. In this problem, the initial speed of the book (3.0 m/s) and its subsequent motion up the slope are analyzed to determine how far it travels before coming to a stop, which is essential for understanding whether it will slide back down.
Recommended video:
Energy Conservation
The principle of energy conservation states that energy cannot be created or destroyed, only transformed from one form to another. In this context, the kinetic energy of the book when it is pushed up the slope is converted into gravitational potential energy as it rises. Analyzing these energy transformations helps determine if the book has enough energy to overcome friction and remain at rest at the highest point.
Recommended video:
Conservation Of Mechanical Energy