Here are the essential concepts you must grasp in order to answer the question correctly.
Newton's Second Law of Motion
Newton's Second Law states that the force acting on an object is equal to the mass of that object multiplied by its acceleration (F = ma). This principle is crucial for understanding how forces interact when the cheerleader lifts another person, as it allows us to calculate the net force required to achieve the specified acceleration.
Recommended video:
Intro to Forces & Newton's Second Law
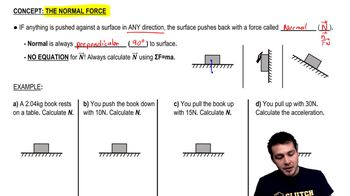
Weight and Normal Force
Weight is the force exerted by gravity on an object, calculated as the product of mass and gravitational acceleration (W = mg). The normal force is the support force exerted by a surface, which in this case is the scale reading. When the cheerleader lifts another person, the normal force changes based on the combined weight and the additional force due to acceleration.
Recommended video:
Net Force
Net force is the total force acting on an object after all the individual forces are combined. In this scenario, when the cheerleader lifts the other cheerleader, the net force will determine how much the scale reads, as it accounts for both the weight of the lifted cheerleader and the additional force required to accelerate her upward.
Recommended video:
Finding Net Forces in 2D Gravitation