Here are the essential concepts you must grasp in order to answer the question correctly.
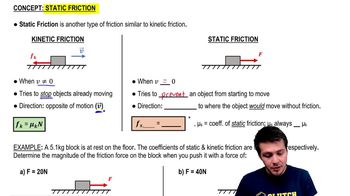
Friction
Friction is the force that opposes the relative motion of two surfaces in contact. In this scenario, the hamster is sliding down the wedge due to the absence of friction (μₛ = μₖ = 0), which means there is no resistance to its motion. Understanding friction is crucial to analyze how the hamster's movement affects the forces acting on the wedge and the spring scale.
Recommended video:
Static Friction & Equilibrium
Newton's Second Law
Newton's Second Law states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass (F = ma). This principle is essential for determining the forces acting on both the hamster and the wedge as the hamster slides down, allowing us to calculate the resultant forces that the spring scale will measure.
Recommended video:
Intro to Forces & Newton's Second Law
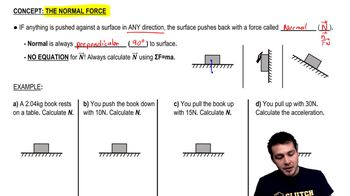
Weight and Normal Force
Weight is the force exerted by gravity on an object, calculated as the product of mass and gravitational acceleration (W = mg). The normal force is the support force exerted by a surface perpendicular to the object resting on it. In this problem, the weight of the hamster and the wedge contributes to the reading on the spring scale, and understanding how these forces interact is key to solving the question.
Recommended video: