Here are the essential concepts you must grasp in order to answer the question correctly.
Acceleration
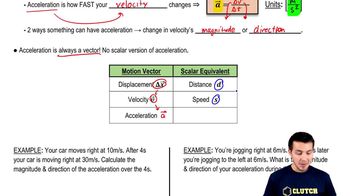
Acceleration is the rate of change of velocity with respect to time. In this context, it can be broken down into components, such as the vertical component (aᵧ) and the horizontal component (aₓ). Understanding how to express acceleration in terms of position and velocity is crucial for analyzing motion along a curved path.
Recommended video:
Velocity Components
Velocity can be decomposed into its components along different axes, typically horizontal (vₓ) and vertical (vᵧ). For a particle moving along a curve, the total velocity is influenced by both components, and their relationship is essential for deriving expressions for acceleration. This concept is fundamental when applying calculus to motion in two dimensions.
Recommended video:
Calculating Velocity Components
Curvilinear Motion
Curvilinear motion refers to the motion of an object along a curved path. In this case, the bead moves along a parabolic wire, which requires the use of parametric equations to describe its position and motion. Understanding the geometry of the path and how it affects the forces and accelerations acting on the object is key to solving the problem.
Recommended video:
 Verified step by step guidance
Verified step by step guidance