Here are the essential concepts you must grasp in order to answer the question correctly.
Free Fall
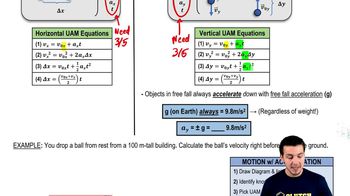
Free fall refers to the motion of an object under the influence of gravity alone, without any air resistance. In the context of the ball bearings, as they fall from the shot tower, they accelerate downward due to gravitational force, which is approximately 9.81 m/s² near the Earth's surface. Understanding free fall is essential to calculate the impact velocity of the bearings when they reach the bottom of the tower.
Recommended video:
Vertical Motion & Free Fall
Kinematic Equations
Kinematic equations describe the motion of objects under constant acceleration. For free-falling objects, the equation v = u + at can be used, where v is the final velocity, u is the initial velocity (zero for a dropped object), a is the acceleration due to gravity, and t is the time of fall. These equations allow us to determine the impact velocity of the ball bearings as they reach the ground.
Recommended video:
Impact Velocity
Impact velocity is the speed at which an object strikes a surface upon falling. It is influenced by the height from which the object falls and the acceleration due to gravity. In this scenario, calculating the impact velocity of the molten metal drops requires knowing the height of the shot tower and applying the principles of free fall and kinematic equations to find the final speed just before they solidify.
Recommended video:
 Verified step by step guidance
Verified step by step guidance