Here are the essential concepts you must grasp in order to answer the question correctly.
Free Fall and Gravitational Acceleration
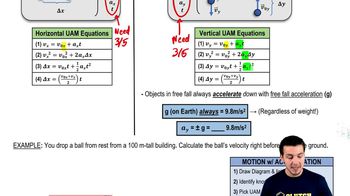
When an object is dropped from a height, it undergoes free fall, accelerating downwards due to gravity at approximately 9.81 m/s². The distance fallen can be calculated using the equation d = 0.5 * g * t², where d is the distance, g is the gravitational acceleration, and t is the time of fall. This concept is crucial for determining how far the lead ball falls before hitting the water.
Recommended video:
Vertical Motion & Free Fall
Constant Velocity in Fluids
Once the lead ball enters the water, it sinks with a constant velocity, meaning it no longer accelerates. This occurs when the forces acting on it, such as buoyancy and drag, balance out the weight of the ball. Understanding this concept is essential for calculating the time it takes for the ball to reach the bottom of the lake after it has entered the water.
Recommended video:
Phase Constant of a Wave Function
Total Distance Calculation
To find the total depth of the lake, one must sum the distance the ball falls through the air and the distance it sinks in the water. The total time of 3.0 seconds is divided into the time of free fall and the time spent sinking, allowing for the calculation of both distances. This concept integrates the previous ideas to provide a complete answer to the question.
Recommended video:
Total Internal Reflection