Here are the essential concepts you must grasp in order to answer the question correctly.
Relative Motion
Relative motion refers to the calculation of the motion of an object as observed from a particular reference point. In this scenario, David is moving at a constant speed while Tina is initially at rest. Understanding relative motion is crucial to determine how fast Tina needs to accelerate to catch up to David.
Recommended video:
Intro to Relative Motion (Relative Velocity)
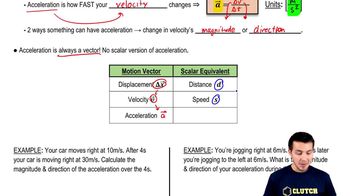
Acceleration
Acceleration is the rate of change of velocity of an object over time. In this case, Tina accelerates at a steady rate of 2.0 m/s². This concept is essential for calculating how her speed increases from rest as time progresses, allowing us to determine her speed when she passes David.
Recommended video:
Kinematic Equations
Kinematic equations describe the motion of objects under constant acceleration. They relate displacement, initial velocity, final velocity, acceleration, and time. To find Tina's speed as she passes David, we can use these equations to calculate her final velocity after a certain time of acceleration from rest.
Recommended video:
 Verified step by step guidance
Verified step by step guidance