Textbook Question
FIGURE EX2.31 shows the acceleration-versus-time graph of a particle moving along the x-axis. Its initial velocity is v0x = 8.0 m/s at t0 = 0 s. What is the particle’s velocity at t = 4.0s? <IMAGE>
299
views
 Verified step by step guidance
Verified step by step guidance
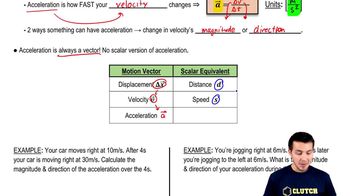


FIGURE EX2.31 shows the acceleration-versus-time graph of a particle moving along the x-axis. Its initial velocity is v0x = 8.0 m/s at t0 = 0 s. What is the particle’s velocity at t = 4.0s? <IMAGE>
FIGURE EX2.5 shows the position graph of a particle. (a) Draw the particle’s velocity graph for the interval . <IMAGE>
Ann and Carol are driving their cars along the same straight road. Carol is located at x = 2.4 mi at t = 0 h and drives at a steady 36 mph. Ann, who is traveling in the same direction, is located at x = 0.0 mi at t = 0.50 h and drives at a steady 50 mph.
c. Draw a position-versus-time graph showing the motion of both Ann and Carol.