Here are the essential concepts you must grasp in order to answer the question correctly.
Projectile Motion
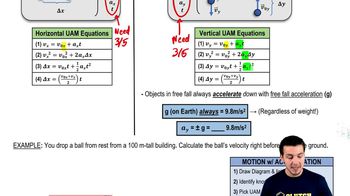
Projectile motion refers to the motion of an object that is launched into the air and is subject to gravitational forces. In this scenario, the first rubber ball is projected upwards with an initial velocity v₀, and its trajectory can be analyzed using kinematic equations to determine its maximum height and the time it takes to reach that height.
Recommended video:
Introduction to Projectile Motion
Free Fall
Free fall describes the motion of an object that is falling solely under the influence of gravity, with no initial velocity. The second rubber ball, dropped from height h, accelerates downwards at a constant rate of 9.81 m/s². Understanding free fall is essential to calculate the position of the second ball at the moment the first ball reaches its peak.
Recommended video:
Vertical Motion & Free Fall
Kinematic Equations
Kinematic equations are mathematical formulas that describe the motion of objects under constant acceleration. They can be used to relate displacement, initial velocity, final velocity, acceleration, and time. In this problem, these equations will help determine the height at which the two balls collide, specifically when the first ball is at its maximum height.
Recommended video:
 Verified step by step guidance
Verified step by step guidance