A certain substance X decomposes. Fifty percent of X remains after 100 minutes. How much X remains after 200 minutes if the reaction order with respect to X is (c) second order?

Key Concepts

Reaction Order

Reaction order refers to the power to which the concentration of a reactant is raised in the rate law of a chemical reaction. It indicates how the rate of reaction depends on the concentration of reactants. For example, a second-order reaction means that the rate is proportional to the square of the concentration of the reactant, which significantly influences how the concentration changes over time.

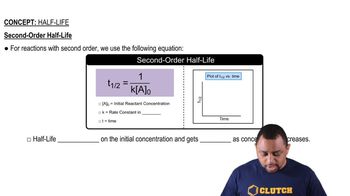
Half-Life in Second-Order Reactions

The half-life of a substance in a second-order reaction is inversely proportional to the initial concentration of the reactant. Unlike first-order reactions, where the half-life is constant, the half-life for second-order reactions increases as the reaction proceeds. This means that as time goes on, it takes longer for the concentration of the reactant to decrease by half.

Integrated Rate Law for Second-Order Reactions

The integrated rate law for a second-order reaction can be expressed as 1/[X] = kt + 1/[X]₀, where [X] is the concentration of the reactant at time t, k is the rate constant, and [X]₀ is the initial concentration. This equation allows us to calculate the concentration of the reactant at any given time, which is essential for determining how much of substance X remains after a specified duration.