The propane fuel (C3H8) used in gas barbeques burns according to the thermochemical equation: C3H8(g) + 5 O2(g) → 3 CO2(g) + 4 H2O(g) ΔH°rxn = –2044 kJ If a pork roast must absorb 1.6×103 kJ to fully cook, and if only 10% of the heat produced by the barbeque is actually absorbed by the roast, what mass of CO2 is emitted into the atmosphere during the grilling of the pork roast?
A 32.5-g iron rod, initially at 22.7 °C, is submerged into an unknown mass of water at 63.2 °C, in an insulated container. The final temperature of the mixture upon reaching thermal equilibrium is 59.5 °C. What is the mass of the water?

Verified Solution
Key Concepts
Thermal Equilibrium

Specific Heat Capacity

Conservation of Energy
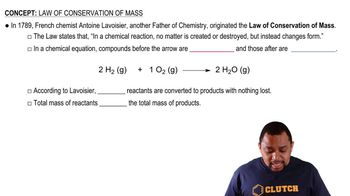
Charcoal is primarily carbon. Determine the mass of CO2 produced by burning enough carbon (in the form of charcoal) to produce 5.00×102 kJ of heat. C(s) + O2(g) → CO2(g) ΔH°rxn = –393.5 kJ
A silver block, initially at 58.5 °C, is submerged into 100.0 g of water at 24.8 °C, in an insulated container. The final temperature of the mixture upon reaching thermal equilibrium is 26.2 °C. What is the mass of the silver block?
A 31.1-g wafer of pure gold, initially at 69.3 °C, is submerged into 64.2 g of water at 27.8 °C in an insulated container. What is the final temperature of both substances at thermal equilibrium?
A 2.85-g lead weight, initially at 10.3 °C, is submerged in 7.55 g of water at 52.3 °C in an insulated container. What is the final temperature of both substances at thermal equilibrium?
Two substances, A and B, initially at different temperatures, come into contact and reach thermal equilibrium. The mass of substance A is 6.15 g and its initial temperature is 20.5 °C. The mass of substance B is 25.2 g and its initial temperature is 52.7 °C. The final temperature of both substances at thermal equilibrium is 46.7 °C. If the specific heat capacity of substance B is 1.17 J/g•°C, what is the specific heat capacity of substance A?
