What mass of natural gas (CH4) must burn to emit 267 kJ of heat? CH4(g) + 2 O2(g) → CO2(g) + 2 H2O(g) ΔH°rxn = –802.3 kJ
The propane fuel (C3H8) used in gas barbeques burns according to the thermochemical equation: C3H8(g) + 5 O2(g) → 3 CO2(g) + 4 H2O(g) ΔH°rxn = –2044 kJ If a pork roast must absorb 1.6×103 kJ to fully cook, and if only 10% of the heat produced by the barbeque is actually absorbed by the roast, what mass of CO2 is emitted into the atmosphere during the grilling of the pork roast?
 Verified step by step guidance
Verified step by step guidance
Verified Solution
Key Concepts
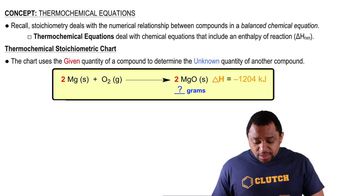
Thermochemical Equations
Heat Transfer Efficiency

Stoichiometry

Nitromethane (CH3NO2) burns in air to produce significant amounts of heat. 2 CH3NO2(l) + 3/2 O2(g) → 2 CO2(g) + 3 H2O(l) + N2(g) ΔH°rxn = –1418 kJ How much heat is produced by the complete reaction of 5.56 kg of nitromethane?
Titanium reacts with iodine to form titanium(III) iodide, emitting heat. 2 Ti(s) + 3 I2(g) → 2 TiI3(s) ΔH°rxn = –839 kJ Determine the mass of titanium that react if 1.55×103 kJ of heat is emitted by the reaction.
Charcoal is primarily carbon. Determine the mass of CO2 produced by burning enough carbon (in the form of charcoal) to produce 5.00×102 kJ of heat. C(s) + O2(g) → CO2(g) ΔH°rxn = –393.5 kJ
A silver block, initially at 58.5 °C, is submerged into 100.0 g of water at 24.8 °C, in an insulated container. The final temperature of the mixture upon reaching thermal equilibrium is 26.2 °C. What is the mass of the silver block?
A 32.5-g iron rod, initially at 22.7 °C, is submerged into an unknown mass of water at 63.2 °C, in an insulated container. The final temperature of the mixture upon reaching thermal equilibrium is 59.5 °C. What is the mass of the water?
