Rank each set of substances in order of increasing standard molar entropy (S°). Explain your reasoning. a. I2(g); F2(g); Br2(g); Cl2(g) b. H2O(g); H2O2(g); H2S(g) c. C(s, graphite); C(s, diamond); C(s, amorphous)
Ch.18 - Free Energy and Thermodynamics
Chapter 18, Problem 55c
Use data from Appendix IIB to calculate ΔS°rxn for each of the reactions. In each case, try to rationalize the sign of ΔS°rxn. c. CO(g) + H2O(g) → H2(g) + CO2(g)
 Verified step by step guidance
Verified step by step guidance1
Identify the reaction: CO(g) + H2O(g) → H2(g) + CO2(g).
Use the formula for the standard entropy change of reaction: ΔS°_rxn = ΣS°_products - ΣS°_reactants.
Look up the standard molar entropy values (S°) for each substance involved in the reaction from Appendix IIB.
Calculate the total entropy of the products: S°(H2) + S°(CO2).
Calculate the total entropy of the reactants: S°(CO) + S°(H2O).
Subtract the total entropy of the reactants from the total entropy of the products to find ΔS°_rxn.
Rationalize the sign of ΔS°_rxn by considering the change in the number of gas molecules and the nature of the substances involved.

Verified Solution
Video duration:
2mWas this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Entropy (ΔS)
Entropy, denoted as ΔS, is a measure of the disorder or randomness in a system. In chemical reactions, it reflects the number of ways the molecules can be arranged. A positive ΔS indicates an increase in disorder, while a negative ΔS suggests a decrease. Understanding how the states of reactants and products influence entropy is crucial for predicting the sign of ΔS°rxn.
Recommended video:
Guided course

Entropy in Thermodynamics
Standard Entropy (S°)
Standard entropy (S°) refers to the absolute entropy of a substance at standard conditions (1 bar and 25°C). Each substance has a specific standard entropy value, which can be found in thermodynamic tables. To calculate ΔS°rxn, the standard entropies of products are subtracted from those of reactants. This concept is essential for quantifying the change in disorder during a reaction.
Recommended video:
Guided course

Standard Molar Entropy
Reaction Direction and Entropy Change
The direction of a chemical reaction and the associated entropy change can often be rationalized by considering the states of the reactants and products. In the given reaction, the conversion of gaseous reactants to gaseous products can lead to an increase in the number of moles of gas, which typically results in a positive ΔS°rxn. Analyzing the molecular complexity and the number of gas molecules helps in predicting the sign of the entropy change.
Recommended video:
Guided course
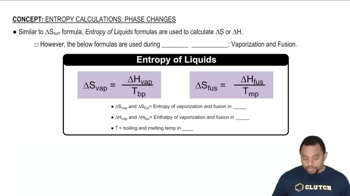
Entropy in Phase Changes
Related Practice
Textbook Question
738
views
Open Question
Use data from Appendix IIB to calculate ΔSrxn ° for each of the reactions. In each case, try to rationalize the sign of ΔSrxn ° . a. C2H4(g) + H2(g) → C2H6(g)
Textbook Question
Use data from Appendix IIB to calculate ΔS°rxn for each of the reactions. In each case, try to rationalize the sign of ΔS°rxn . b. C(s) + H2O(g) → CO(g) + H2(g)
2216
views
1
rank
Textbook Question
Use data from Appendix IIB to calculate ΔS°rxn for each of the reactions. In each case, try to rationalize the sign of ΔS°rxn. d. 2 H2S(g) + 3 O2(g) → 2 H2O(l) + 2 SO2(g)
347
views
Textbook Question
Use data from Appendix IIB to calculate ΔS°rxn for each of the reactions. In each case, try to rationalize the sign of ΔS°rxn . a. 3 NO2(g) + H2O(l) → 2 HNO3(aq) + NO(g)
479
views
Textbook Question
Use data from Appendix IIB to calculate ΔS°rxn for each of the reactions. In each case, try to rationalize the sign of ΔS°rxn. b. Cr2O3(s) + 3 CO(g) → 2 Cr(s) + 3 CO2(g)
669
views
