Here are the essential concepts you must grasp in order to answer the question correctly.
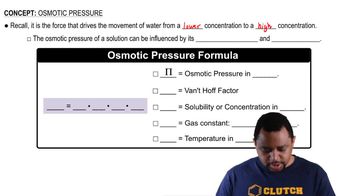
Osmotic Pressure
Osmotic pressure is the pressure required to prevent the flow of solvent into a solution through a semipermeable membrane. It is directly proportional to the concentration of solute particles in the solution, as described by the formula π = iCRT, where π is osmotic pressure, i is the van 't Hoff factor, C is the molar concentration, R is the ideal gas constant, and T is the temperature in Kelvin.
Recommended video:
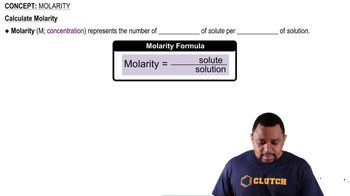
Molarity and Concentration
Molarity is a measure of concentration defined as the number of moles of solute per liter of solution. To calculate the molarity of the insulin solution, one must first convert the mass of insulin into moles using its molar mass, then divide by the volume of the solution in liters. This concentration is essential for determining the osmotic pressure.
Recommended video:
Hydrostatic Pressure and Height of Water Column
Hydrostatic pressure is the pressure exerted by a fluid at equilibrium due to the force of gravity. The height of a water column can be calculated using the formula P = ρgh, where P is the pressure, ρ is the density of the fluid, g is the acceleration due to gravity, and h is the height. In this context, the osmotic pressure calculated will be converted to the height of a mercury column using the density of mercury.
Recommended video: