Textbook Question
Calculate the mass defect (in g/mol) and the binding energy (in MeV/nucleon) for the following nuclei. Which of the two is more stable?
(a) 7Li (atomic mass = 7.016004)
(b) 39K (atomic mass = 38.963706)
533
views
 Verified step by step guidance
Verified step by step guidance
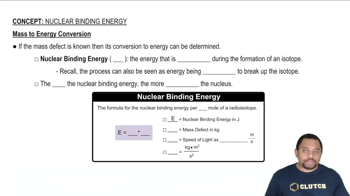


Calculate the binding energy (in MeV/nucleon) for the following nuclei. (a)58Ni (atomic mass = 57.93535)
Calculate the binding energy (in MeV/nucleon) for the following nuclei. (b) 84Kr (atomic mass = 83.91151)
The radioactive isotope 100Tc decays to form the stable iso-tope 100Mo. (a) There are two possible pathways for this decay. Write balanced equations for both.
The radioactive isotope 100Tc decays to form the stable iso-tope 100Mo. (b) Only one of the pathways is observed. Calculate the energy released by both pathways, and explain why only one is observed. Relevant masses are: 100Tc = 99.907 657, 100Mo = 99.907 48, electron = 0.000 548 6.