Calculate the binding energy (in MeV/nucleon) for the following nuclei. (b) 84Kr (atomic mass = 83.91151)

Verified step by step guidance

1. Identify the number of protons and neutrons in the nucleus of \( ^{84}\text{Kr} \). Krypton (Kr) has an atomic number of 36, so it has 36 protons. The number of neutrons is calculated by subtracting the number of protons from the mass number: \( 84 - 36 = 48 \) neutrons.
2. Calculate the total mass of the protons and neutrons if they were free particles. Use the mass of a proton (approximately 1.00728 u) and the mass of a neutron (approximately 1.00866 u). Multiply the mass of a proton by the number of protons and the mass of a neutron by the number of neutrons, then sum these values.
3. Determine the mass defect by subtracting the actual atomic mass of \( ^{84}\text{Kr} \) (given as 83.91151 u) from the total mass of the free protons and neutrons calculated in the previous step.
4. Convert the mass defect from atomic mass units (u) to energy in mega-electronvolts (MeV) using Einstein's equation \( E = \Delta m c^2 \), where \( c^2 \) is approximately 931.5 MeV/u.
5. Calculate the binding energy per nucleon by dividing the total binding energy (in MeV) by the total number of nucleons (84 in this case).

Key Concepts

Binding Energy

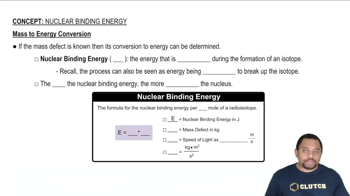
Binding energy is the energy required to disassemble a nucleus into its constituent protons and neutrons. It reflects the stability of a nucleus; a higher binding energy indicates a more stable nucleus. This energy can be calculated using the mass defect, which is the difference between the mass of the individual nucleons and the mass of the nucleus itself.

Mass Defect

The mass defect is the difference between the total mass of the separate nucleons and the actual mass of the nucleus. This discrepancy arises because some mass is converted into energy when nucleons bind together, according to Einstein's equation E=mc². The mass defect is crucial for calculating the binding energy of a nucleus.

MeV/nucleon

MeV/nucleon is a unit of measurement that expresses binding energy per nucleon in mega-electronvolts. This unit allows for easy comparison of the binding energies of different nuclei, as it normalizes the energy value to the number of nucleons present. Understanding this unit is essential for interpreting the stability and energy characteristics of various isotopes.