The thermite reaction, Fe2O31s2 + 2 Al1s2 ¡2 Fe1s2 + Al2O31s2, H = -851.5 kJ>mol, is one of the most exothermic reactions known. Because the heat released is sufficient to melt the iron product, the reaction is used to weld metal under the ocean. How much heat is released per mole of Al2O3 produced? How does this amount of thermal energy compare with the energy released when 2 mol of protons and 2 mol of neutrons combine to form 1 mol of alpha particles?
The atomic masses of hydrogen-2 (deuterium), helium-4, and lithium-6 are 2.014102 amu, 4.002602 amu, and 6.0151228 amu, respectively. For each isotope, calculate
(c) the nuclear binding energy per nucleon.
 Verified step by step guidance
Verified step by step guidance
Verified Solution
Key Concepts
Nuclear Binding Energy
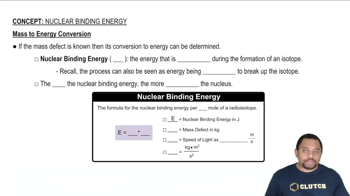
Binding Energy per Nucleon

Mass Defect

How much energy must be supplied to break a single aluminum-27 nucleus into separated protons and neutrons if an aluminum-27 atom has a mass of 26.9815386 amu? How much energy is required for 100.0 g of aluminum-27? (The mass of an electron is given on the inside back cover.)
The atomic masses of nitrogen-14, titanium-48, and xenon-129 are 13.999234 amu, 47.935878 amu, and 128.904779 amu, respectively. For each isotope, calculate (a) the nuclear mass.
Based on the following atomic mass values: 1H, 1.00782 amu; 2H, 2.01410 amu; 3H, 3.01605 amu; 3He, 3.01603 amu; 4He, 4.00260 amu—and the mass of the neutron given in the text, calculate the energy released per mole in each of the following nuclear reactions, all of which are possibilities for a controlled fusion process:
(a) 21H + 31H → 42He + 10n
(b) 21H + 21H → 32He + 10n
(c) 21H + 32He → 42He + 11H
