The atomic masses of hydrogen-2 (deuterium), helium-4, and lithium-6 are 2.014102 amu, 4.002602 amu, and 6.0151228 amu, respectively. For each isotope, calculate
(c) the nuclear binding energy per nucleon.
 Verified step by step guidance
Verified step by step guidance
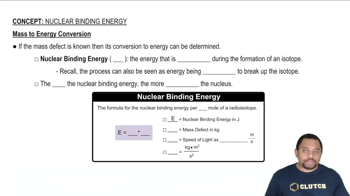


The atomic masses of hydrogen-2 (deuterium), helium-4, and lithium-6 are 2.014102 amu, 4.002602 amu, and 6.0151228 amu, respectively. For each isotope, calculate
(c) the nuclear binding energy per nucleon.
The atomic masses of nitrogen-14, titanium-48, and xenon-129 are 13.999234 amu, 47.935878 amu, and 128.904779 amu, respectively. For each isotope, calculate (a) the nuclear mass.
Iodine-131 is a convenient radioisotope to monitor thyroid activity in humans. It is a beta emitter with a half-life of 8.02 days. The thyroid is the only gland in the body that uses iodine. A person undergoing a test of thyroid activity drinks a solution of NaI, in which only a small fraction of the iodide is radioactive. (c) A normal thyroid will take up about 12% of the ingested iodide in a few hours. How long will it take for the radioactive iodide taken up and held by the thyroid to decay to 0.01% of the original amount?