Textbook Question
Consider the unit cells shown here for three different structures that are commonly observed for metallic elements. (b) Which structure(s) corresponds to the least dense packing of atoms?
453
views
 Verified step by step guidance
Verified step by step guidance

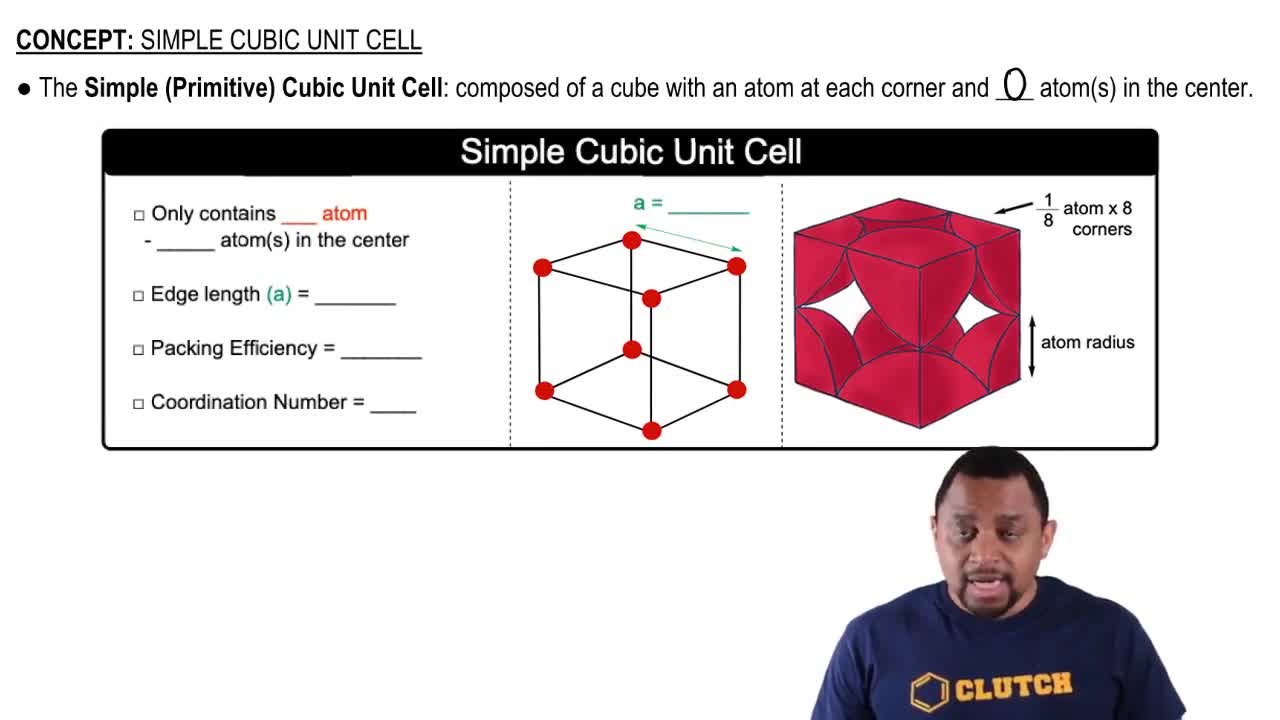

Consider the unit cells shown here for three different structures that are commonly observed for metallic elements. (b) Which structure(s) corresponds to the least dense packing of atoms?
Sodium metal (atomic weight 22.99 g>mol) adopts a body-centered cubic structure with a density of 0.97 g>cm3. (b) If sodium didn't react so vigorously, it could float on water. Use the answer from part (a) to estimate the density of Na if its structure were that of a cubic close-packed metal. Would it still float on water?