Figure 7.4 shows the radial probability distribution functions for the 2s orbitals and 2p orbitals. (b) How would you modify Slater's rules to adjust for the difference in electronic penetration of the nucleus for the 2s and 2p orbitals?
 Verified step by step guidance
Verified step by step guidance
Verified Solution
Key Concepts
Radial Probability Distribution

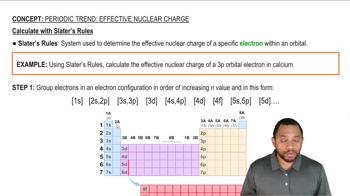
Slater's Rules
Electron Penetration

Consider the stable elements through lead (Z = 82). In how many instances are the atomic weights of the elements out of order relative to the atomic numbers of the elements?
Figure 7.4 shows the radial probability distribution functions for the 2s orbitals and 2p orbitals. (a) Which orbital, 2s or 2p, has more electron density close to the nucleus?
(a) If the core electrons were totally effective at screening the valence electrons and the valence electrons provided no screening for each other, what would be the effective nuclear charge acting on the 3s and 3p valence electrons in P?
(b) Repeat these calculations using Slater’s rules.
(c) Detailed calculations indicate that the effective nuclear charge is 5.6+ for the 3s electrons and 4.9+ for the 3p electrons. Why are the values for the 3s and 3p electrons different?
