Here are the essential concepts you must grasp in order to answer the question correctly.
Factoring Quadratic Equations
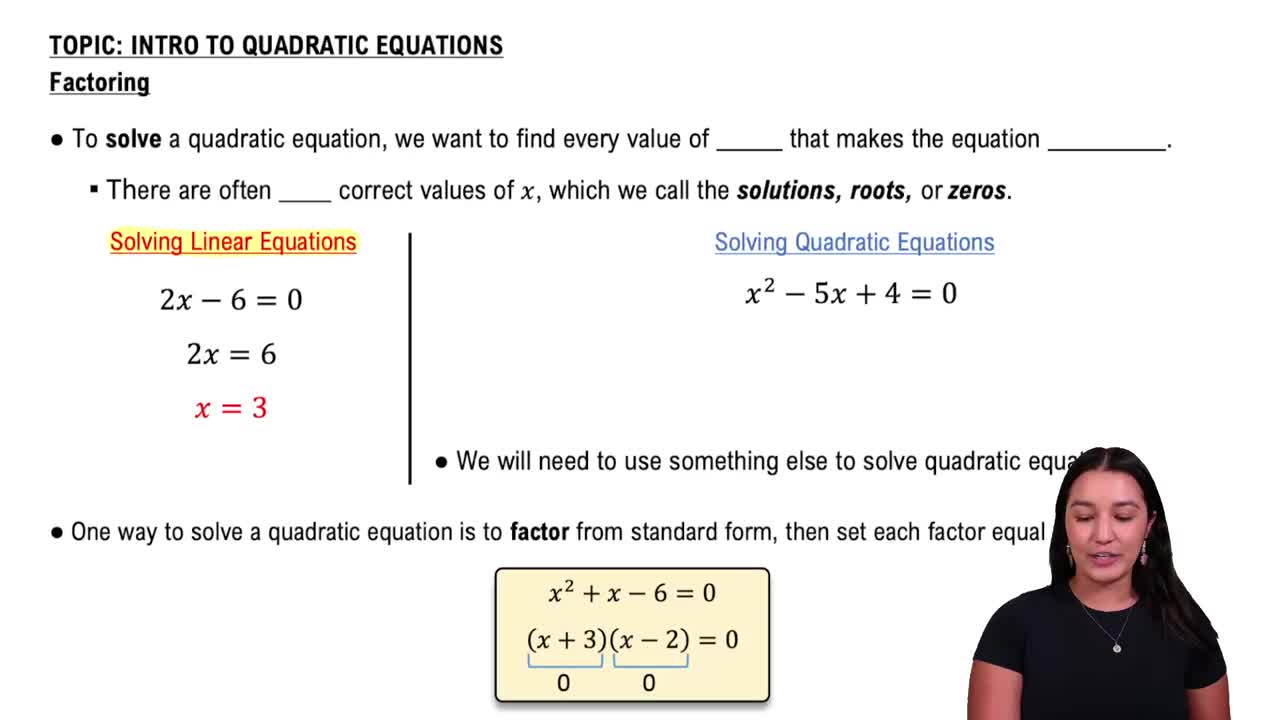
Factoring is a method used to solve quadratic equations by expressing them as a product of their linear factors. In the case of the equation 3x^2 + 12x = 0, we can factor out the greatest common factor, which simplifies the equation and allows us to find the values of x that satisfy it.
Recommended video:
Solving Quadratic Equations by Factoring
Zero Product Property
The Zero Product Property states that if the product of two factors equals zero, at least one of the factors must be zero. This principle is crucial when solving factored equations, as it allows us to set each factor equal to zero to find the solutions for the variable.
Recommended video:
Product, Quotient, and Power Rules of Logs
Greatest Common Factor (GCF)
The Greatest Common Factor is the largest factor that divides two or more numbers. In the equation 3x^2 + 12x, the GCF is 3x, which can be factored out to simplify the equation. Identifying the GCF is an essential step in the factoring process, making it easier to solve the equation.
Recommended video:
Graphs of Common Functions