Here are the essential concepts you must grasp in order to answer the question correctly.
Polynomial Inequalities
Polynomial inequalities involve expressions that compare a polynomial to a value, typically zero, using inequality symbols such as ≥, ≤, >, or <. To solve these inequalities, one must find the values of the variable that make the inequality true, often by determining the roots of the polynomial and testing intervals between these roots.
Recommended video:
Interval Notation
Interval notation is a mathematical notation used to represent a range of values on the real number line. It uses parentheses and brackets to indicate whether endpoints are included (closed interval) or excluded (open interval). For example, the interval [a, b) includes 'a' but not 'b', while (a, b) excludes both endpoints.
Recommended video:
Graphing Solution Sets
Graphing solution sets on a real number line visually represents the values that satisfy the inequality. This involves marking the critical points (roots) and shading the appropriate regions based on the inequality's direction. Understanding how to accurately depict these solutions helps in interpreting the results of polynomial inequalities.
Recommended video:
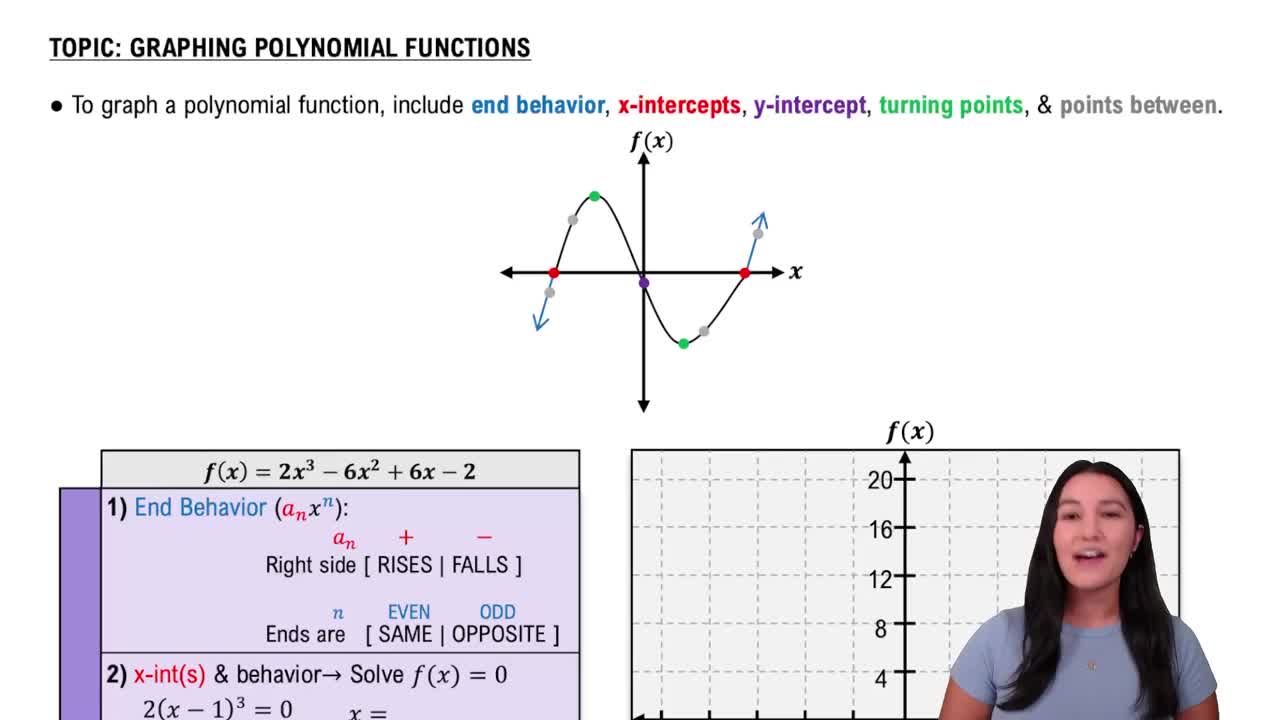
Graphing Polynomial Functions
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution